Full-Length PSAT Math Practice Test-Answers and Explanations

27- Choice D is correct
Percent of cities in the type of pollution A: \(\frac{8}{10}×100=80\%\)
Percent of cities in the type of pollution C: \(\frac{7}{10}×100=70\%\)
Percent of cities in the type of pollution E: \(\frac{5}{10}×100=50\%\)

28- Choice D is correct
Let x be the number of cities that need to be added to the type of pollution E. Then:
\(\frac{x+5}{9}=0.8→x+5=9×0.8→x+5=7.2→x=2.2\)

29- Choice B is correct
Since \(f(x)\) is a linear function with a negative slope, then when \(x=-4,f(x)\) is maximum and when \(x=5,f(x)\) is minimum. Then the ratio of the minimum value to the maximum value of the function is: \(\frac{f(5)}{f(-4)}=\frac{-2(5)+2}{-2(-4)+2}=\frac{-8}{10}=\frac{-4}{5}\)

30- Choice B is correct
AB=15 And BC=36, AC=\(\sqrt{15^2+36^2} =\sqrt{225+1296}=\sqrt{1521}=39\)
Perimeter =\(15+36+39=90\), Area =\(\frac{36×15}{2}=270\)
In this case, the ratio of the perimeter of the triangle to its area is: \(\frac{90}{270}=\frac{1}{3}\)
If the sides AB and AC become one-third shorter, then: AB=5 And AC=13
BC=\(\sqrt{13^2-5^2}=\sqrt{169-25}=\sqrt{144}=12\), Perimeter =\(5+12+13=30\)
Area =\9\frac{12×5}{2}=30\), In this case, the ratio of the perimeter of the triangle to its area is: \(\frac{30}{30}=1\)

31- Choice B is correct
The ratio of boys to girls is 5:9. Therefore, there are 5 boys out of 14 students. To find the answer, first, divide the total number of students by 14, then multiply the result by 5.
\(84 ÷ 14 = 6 ⇒ 5 × 6 = 30\), There are 30 boys and \(54 (84 – 30)\) girls. So, 24 more boys should be enrolled to make the ratio 1:1

32- Choice A is correct
Ratio of women to men in city A: \(\frac{400}{455}=0.88\)
Ratio of women to men in city B: \(\frac{620}{868}=0.71\)
Ratio of women to men in city C: \(\frac{600}{700}=0.86\)
Ratio of women to men in city D: \(\frac{650}{800}=0.81\)
Choice A provides the maximum ratio of women to men in the four cities.

33- Choice A is correct
Percentage of men in city A = \(\frac{455}{855}×100=56.87\%\)
Percentage of women in city C = \(\frac{600}{1300}×100=46.15\%\)
Percentage of men in city A to percentage of women in city C = \\(frac{56.87}{46.15}=1.23\)

34- Choice A is correct
Let the number of women should be added to city D be \(x\), then:
\(\frac{650+x}{800}=1.4→650+x=800×1.4=1120→x=470\)

35- Choice C is correct
If \(f(x)=-2x+2(2x+3)+1\), then find \(f(-3x)\) by substituting \(4x\) for every \(x\) in the function. This gives: \(f(-3x)=-2(-3x)+2(2(-3x)+3)+1=6x-12x+6+1\),
It simplifies to: \(f(-3x)=6x-12x+6+1=-6x+7\)

36- Choice C is correct
The amount of petrol consumed after \(x\) hours is: \(7.5×x=7.5x\)
Petrol remaining after x hours driving: \(60-7.5x\)

37- Choice A is correct
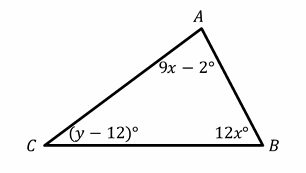
In the figure angle A is labeled \((9x-2)\) and it measures 43. Thus, \(9x-2=43\) and \(9x=45\) or \(x=5\). That means that angle B, which is labeled \((12x)\), must measure \(12×5=60\).
Since the three angles of a triangle must add up to 180, \(43+60+y-12=180\), then: \(y+91=180→y=180-91=89\)

38- Choice A is correct
??????? (????) \(= \frac{sum \space of \space terms}{number \space of \space terms}=\frac{8+12+15+15+8+14}{6}=12\)

39- Choice D is correct
The perimeter of the rectangle is: \(2x+2y=40→x+y=20→x=20-y\)
The area of the rectangle is: \(x×y=96→(20-y)(y)=96→y^2-20y+96=0\)
Solve the quadratic equation by factoring method.
\((y-8)(y-12)=0→y=8\) (Unacceptable, because y must be greater than 10) or \(y=12\)
If \(y=12 →x×y=96→x×12=96→x=8\)

40- Choice D is correct
The equation \(\frac{a-b}{2b}=\frac{5}{9}\) can be rewritten as \(\frac{a}{2b}-\frac{b}{2b}=\frac{5}{9}\), from which it follows that \(\frac{a}{2b}-\frac{1}{2}=\frac{5}{9}\), or \(\frac{a}{2b}=\frac{5}{9}+\frac{1}{2}=\frac{19}{18}.\)⇒\(\frac{a}{b}=\frac{19}{9}\), Subtract 12 from both sides of the equation. Then: \(x+12=10→x=-2\)

41- Choice D is correct
\(Cos⁡β=\frac{Adjacent \space side}{hypotenuse}\), To find the hypotenuse, we need to use the Pythagorean theorem.
\(a^2+b^2=c^2→c=\sqrt{a^2+b^2}, cos (β)=\frac{a}{c}=\frac{a}{\sqrt{a^2+b^2}}, sec(β)=\frac{1}{cos ⁡(β)} =\frac{\sqrt{a^2+b^2 }}{a}\)

42- Choice B is correct
\(|\frac{2x}{3}-4x-6|<4.⇒-4<\frac{2x}{3}-4x-6<4\).Multiply both sides by 3. ⇒ \(-12<2x-12x-18<12\), and add 18 from all sides of the inequality. \(18-12<-10x-18+18<12+18⇒6<-10x<30\), Divide all sides by \(-10\). \(\frac{6}{-10}>x>-3\)
(Remember that when you divide all sides of an inequality by a negative number, the inequality sign will be swapped. < becomes >)

43- Choice D is correct
\(x\) is directly proportional to the square of \(y\). Then: \(x=cy^2
18=c(3)^2→18=9c→c=18/9=2\), The relationship between \(x\) and \(y\) is: \(x=2y^2
x=288, 288=2y^2→y^2=288/2=144→y=12\)

44- Choice B is correct
\(\begin{cases}2x+4y=6 \\x+3y=-20 \end{cases}\)
Multiply the second equation by \(-2\) then,
\(\begin{cases}2x+4y=6 \\-2x-6y=40 \end{cases}\)
Add two equations, \(-2y=46→y=-23\), plug in the value of y into the second equation, \(x+3y=-20→x+4(-23)=-20→x=49\)
Subtract 12 from both sides of the equation. Then: \(x+12=10→x=-2\)

45- The answer is 6
\(x+4y=\frac{-8y^2+12}{2x}\), Multiply both sides by \(2x\).
\(2x×(x+4y)=2x×((\frac{-8y^2+12}{2x})→2x^2+8xy=-8y^2+12
→2x^2+8xy+8y^2=12→2×(x^2+4xy+4y^2 )=12→x^2+4xy+4y^2=6
x^2+4xy+4y^2=(x+2y)^2, Then: →(x+2y)^2=6\)

46- The answer is 14.14
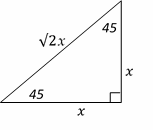
The relationship among all sides of special right triangle
\(45^\circ-45^\circ- 90^\circ\) is provided in this triangle: According to picture length of ladder is \(\sqrt{2} x⇒L=10\sqrt{2}=14.14\)

47- The answer is \(\frac{3}{2}\)
Let x be the length of an edge of cube, then the volume of a cube is: \(V=x^3\)
The surface area of cube is: \(SA=6x^2\), The volume of cube A is \(\frac{1}{4}\) of its surface area. Then:
\(x^3=\frac{6x^2)}{4}→x^3=\frac{3}{2}x^2\), divide both side of the equation by \(x^2\). Then: \(\frac{x^3}{x^2} =\frac{3x^2}{2x^2} →x=\frac{3}{2}\)

48- The answer is \(\frac{19}{12}\)
The intersection of two functions is the point with 3 for \(x\). Then:
\(f(3)=g(3) and g(3)=(3+2)=5\),
Then, \(f(2)=5→a(3)^2+b(3)+c=5.⇒9a+3b+c=5\) (i)
The value of x in the vertex of the parabola is: \(x=-\frac{b}{2a}→-3=-\frac{b}{2a}→b=6a\) (ii)
In the point \((-3, 11)\), the value of the \(f(x)\) is 11.
\(f(-3)=11→a(-3)^2+b(-3)+c=11→9a-3b+c=11\) (iii)
Using the first two equation: \(\begin{cases}9a+3b+c=5 \\ 9a-3b+c=11 \end{cases} \)
Equation 1 minus equation 2 is: (i)-(iii) →\(6b=-6→b=-1\) (iv)
Plug in the value of b in the second equation: \9b=6a →a=\frac{b}{6}=-\frac{1}{6}\)
Plug in the values of a and be in the first equation. Then:
\(9(-\frac{1}{6})+3(-1)+c=5⇒c=5+3+\frac{9}{6}=\frac{57}{6}=9 \frac{1}{2}\)
The product of a, b and c\(=(-\frac{1}{6})×(-1)×\frac{57}{6}=\frac{57}{36}=\frac{19}{12}\)

College Entrance Tests

The Best Books to Ace the PSAT Math Test

PSAT Math in 10 Days The Most Effective PSAT Math Crash Course

Download

$29.99 Original price was: $29.99.$16.99Current price is: $16.99.