How to Write Inequalities from Number Lines?

A Step-by-step Guide to Writing Inequalities from Number Lines

Writing inequalities from number lines involves identifying the set of numbers that satisfy the inequality represented by the number line. Here are the steps to follow:

Step 1: Identify the endpoints of the number line:

Look at the endpoints of the number line and determine whether they are open or closed circles.

A closed circle represents an endpoint that is included in the solution set, while an open circle represents an endpoint that is not included.

Step 2: Write the inequality symbol:

Depending on whether the endpoint is included or not, write the appropriate inequality symbol.

For a closed endpoint, use the symbol \(“≤”\) or \(“≥”\), which means “less than or equal to” or “greater than or equal to”, respectively.

For an open endpoint, use the symbol \(“<“\) or \(“>”\) which means “less than” or “greater than”.

Step 3: Determine the direction of the inequality:

Look at the direction in which the number line extends. If it extends to the right, use the symbol \(“>”\), and if it extends to the left, use the symbol \(“<“\).

Step 4: Combine the symbols:

Combine the inequality symbol with the direction symbol to create the final inequality.

For example, if the endpoint is closed and the number line extends to the right, the inequality would be written as \(“x ≥ a”\), where \(“a”\) is the value of the endpoint.

If the endpoint is open and the number line extends to the left, the inequality would be written as \(“x < b”\), where \(“b”\) is the value of the endpoint.

Writing Inequalities from Number Lines – Examples 1

What inequality does this number line show?

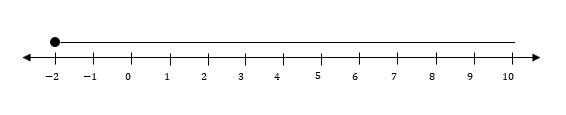
Solution:

In this example, the endpoint is a closed circle at \(-2\), and the number line extends to the right. This means that the inequality is \(“x ≥ -2″\).

Writing Inequalities from Number Lines – Examples 2

What inequality does this number line show?

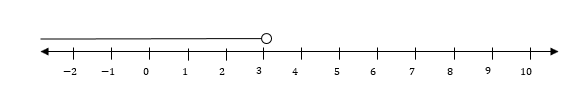
Solution:

In this example, the endpoint is an open circle at \(3\), and the number line extends to the left. This means that the inequality is \(“x < 3″\).

Exercises for Writing Inequalities from Number Lines

What inequality does each number line show?

1.

2.

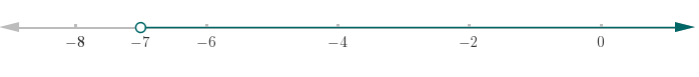
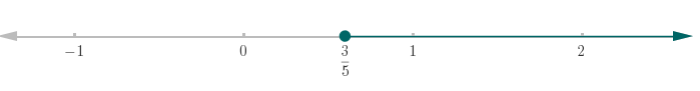
3.

1. \(\color{blue}{x\le 15}\)
2. \(\color{blue}{x>-7}\)
3. \(\color{blue}{x\ge \frac{3}{5}}\)