How to Find the Volume of Spheres? (+FREE Worksheet!)

In this article, you will learn how to find volumes of Spheres in a few simple steps.

Related Topics

• How to Solve Pythagorean Theorem Problems
• How to Solve Triangles Problems
• How to Find the Perimeter of Polygons
• How to Calculate the Area of Trapezoids
• How to Find the Volume of Cones and Pyramids

Step by step guide to finding volume of spheres

A sphere is a completely round three-dimensional object like a ball or a globe. This geometric volume can be specified by a set of all points located at a distance \(r\) (radius) from a point (center). The sphere is perfectly symmetrical and has no edges or vertices.

To find the volume of the sphere, we need to have the radius of the sphere, then we can use the following relation:

Sphere volume: \(=\frac{4}{3}\)\(\times\)Pi number\(\times\)radius\(\times\)radius\(\times\)radius

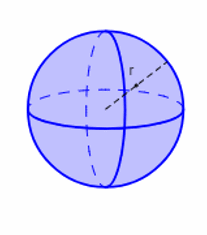
If we want to write the above relation using mathematical symbols, we will have:

\(V=\frac{4}{3}\times π\times r^3\)

In fact, in this relation \(V\) represents the volume of the sphere, and \(r\) symbolizes the radius of the sphere in question.

Finding Volume of Spheres – Example 1:

Find the volume of a sphere whose radius is \(5 cm\). \((π=3.14)\)

Solution: Given: radius, \(r=5 cm\)

The volume of a sphere formula: \(V=\frac{4}{3}\times π\times r^3\)

\(r=5 cm→V=\frac{4}{3}\times π\times r^3=\frac{4}{3}\times 3.14\times (5)^3=523.33 cm^3\)

Finding Volume of Spheres – Example 2:

Find the volume of a sphere whose diameter is \(22 cm\). \((π=3.14)\)

Solution: Given, diameter: \(22 cm\)

Then: radius \(=\frac{diameter}{2}=\frac{22cm}{2}=11 cm\)

The volume of a sphere formula: \(V=\frac{4}{3}\times π\times r^3\)

\(r=11 cm→V=\frac{4}{3}\times π\times r^3=\frac{4}{3}\times 3.14\times (11)^3=5,572.45 cm^3\)

Finding Volume of Spheres – Example 3:

Find the volume of a sphere whose radius is \(2 ft\). \((π=3.14)\)

Solution: Given: radius, \(r=2 ft\)

The volume of a sphere formula: \(V=\frac{4}{3}\times π\times r^3\)

\(r=2 ft→V=\frac{4}{3}\times π\times r^3=\frac{4}{3}\times 3.14\times (2)^3=33.49 ft^3\)

Finding Volume of Spheres – Example 4:

Find the volume of a sphere whose diameter is \(50 ft\). \((π=3.14)\)

Solution: Given, diameter: \(50 ft\)

Then: radius \(=\frac{diameter}{2}=\frac{50ft}{2}=25 ft\)

The volume of a sphere formula: \(V=\frac{4}{3}\times π\times r^3\)

\(r=25 ft→V=\frac{4}{3}\times π\times r^3=\frac{4}{3}\times 3.14\times (25)^3=65,416.67 ft^3\)

Exercises for Finding Volume of Spheres

Find the volume of each sphere. \((π=3.14)\)

1. \(\color{blue}{radius=3.5 ft}\)
2. \(\color{blue}{diameter=13 cm}\)
3. \(\color{blue}{radius=13 cm}\)
4. \(\color{blue}{diameter=14 ft}\)
5. \(\color{blue}{radius=19 ft}\)
6. \(\color{blue}{diameter=54 ft}\)

1. \(\color{blue}{V=179.5 ft^3}\)
2. \(\color{blue}{V=1,149.76 cm^3}\)
3. \(\color{blue}{V=9,198.11 cm^3}\)
4. \(\color{blue}{V=1,436.03 ft^3}\)
5. \(\color{blue}{V=28,716.35 ft^3}\)
6. \(\color{blue}{V=82,406.16 ft^3}\)