How to Find the Volume and Surface Area of a Triangular Pyramid?

A three-dimensional shape whose four sides are triangular is known as a triangular pyramid. Triangular pyramids are irregular, regular, and right-angled.

Related Topics

• How to Find the Surface Area of a Pyramid?

A step-by-step guide to finding the volume and surface area of the triangular pyramid

A triangular pyramid is a three-dimensional shape in which all faces are triangular. It is a pyramid with a triangular base connected by four triangular faces in which \(3\) faces meet at a vertex. If it is a right triangular pyramid, the base is a right-angled triangle and the other sides of the triangle are isosceles.

Types of the triangular pyramid

Like any other geometric shape, triangular pyramids can be classified into regular and irregular pyramids.

Regular triangular pyramid

A regular triangular pyramid has equilateral triangles. Since it is made of equilateral triangles, its interior angles are \(60\) degrees.

Irregular triangular pyramid

Irregular triangular pyramids also have triangular faces, but they are not equilateral. The internal angles in each plane add up to \(180°\) due to the triangle. Unless a triangular pyramid is mentioned explicitly as irregular, all triangular pyramids are assumed to be regular triangular pyramids.

Right triangular pyramid

The right triangular pyramid has the base of the right triangle and its apex is located above the center of the base. It has \(1\) right-angled base, \(6\) edges, \(3\) triangular faces, and \(4\) vertices.

Properties of a triangular pyramid

A triangular pyramid feature helps us identify a pyramid from a set of specific shapes quickly and easily. The different properties of a triangular pyramid are:

• A triangular pyramid has \(4\) triangular faces, \(6\) edges, and \(4\) vertices.
• \(3\) edges meet at each of its vertexes.
• A triangular pyramid has no parallel faces.
• A regular triangular pyramid has equilateral triangles for all its faces. It has \(6\) planes of symmetry.
• Triangular pyramids can be regular, irregular, and right-angled.

Triangular pyramid formulas

There are various formulas used to calculate the volume and area of triangular pyramids. See the following figure to relate to the formulas given below:

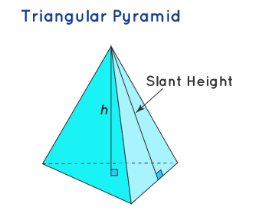
We can calculate the volume of a triangular pyramid with this formula:

\(\color{blue}{Volume\:of\:Triangular\:Pyramid\:=\:\frac{1}{3}\:×\:Base\:Area\:×\:Height}\)

Where we multiply the area of the triangular base by the height of the pyramid (measured from the base to up) and then divide the result by \(3\) according to the formula.

We can calculate the total area of a triangular pyramid by this formula:

\(\color{blue}{Total\:Surface\:area\:of\:a\:Triangular\:Pyramid\:=\:Base\:Area\:+\:\frac{1}{2}\:\left(Perimeter\:of\:the\:base\:×\:Slant\:Height\right)}\)

Geometry for Beginners The Ultimate Step by Step Guide to Acing Geometry

Download

$27.99 Original price was: $27.99.$17.99Current price is: $17.99.

where slant height is the distance from its triangular face along the center of the face to the apex.

Finding the Volume and Surface Area of the Triangular Pyramid – Example 1:

Find the volume of a triangular pyramid having a base area of \(15\space cm^2\) and a height of \(6\space cm\).

Solution:

Using this formula to find the volume of a triangular pyramid:

\(Volume=\frac{1}{3}×\:Base\:area\:×\:Height\)

\(= \frac{1}{3}× 15 × 6\)

\(=\frac{90}{3}\)

\(= 30\space cm^3\)

Exercises for Finding the Volume and Surface Area of the Triangular Pyramid

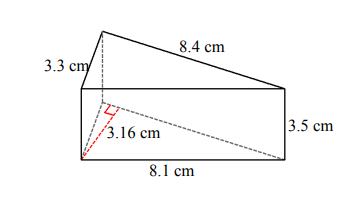
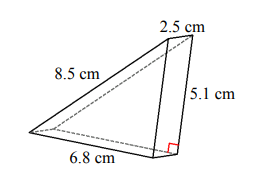
Find the volume and surface area for each triangular pyramid.

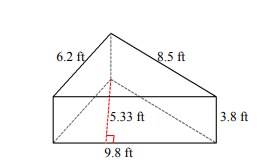
• \(\color{blue}{V= 99.2\space ft^3, A= 145.3\space ft^2}\)
• \(\color{blue}{V= 46.5 \space cm^3, A=95.8\space cm^2}\)
• \(\color{blue}{V=43.4 \space cm^3, A=85.7\space cm^2}\)

Frequently Asked Questions

What is a triangular pyramid?

A 3D shape with a triangular base and three triangular faces meeting at a single apex point. It has 4 vertices, 6 edges, and 4 faces.

What is the formula for the volume of a triangular pyramid?

V = (1/3) * base area * height, where height is the perpendicular distance from the apex to the plane of the base.

Walk through a volume calculation.

Base triangle with base 6 and height 4: base area = (1/2)(6)(4) = 12. If pyramid height = 9: V = (1/3)(12)(9) = 36 cubic units.

How do I find the surface area?

Add the area of the base to the areas of the three slant faces. Each slant face is itself a triangle with a known base (a side of the base triangle) and slant height.

What is the slant height?

The height of one of the slant faces, measured from the apex perpendicular to the base edge of that face — NOT the perpendicular height of the pyramid itself.

What is a regular tetrahedron?

A triangular pyramid where all four faces are congruent equilateral triangles. All edges are equal in length.

How is the volume of a regular tetrahedron with edge a?

V = a^3 sqrt(2) / 12. A regular tetrahedron with edge 6 has V = 216 sqrt(2) / 12 = 18 sqrt(2) ≈ 25.46 cubic units.

Why is the volume one-third of a prism with the same base and height?

Because three congruent pyramids can be assembled to fill a prism with the same base and height — a result you can prove with calculus integration or with a geometric assembly argument.

Where are triangular pyramids used?

Pyramid roof designs, prism-shaped containers, molecular geometry (methane CH4 has a tetrahedral shape), and crystal lattices.

What grade is this topic for?

Often introduced in high school geometry. Volume formulas for prisms and pyramids appear in middle school; the triangular pyramid often appears as a special case.

Related Lessons You May Like

• How to find the area of triangles
• How to find volume and surface area of cubes
• How to calculate the volume of cubes and prisms
• Volume of cylinders and spheres
• How to use the Pythagorean Theorem

For a workbook on geometry, Geometry for Beginners walks every topic from first principles. Pre-Algebra for Beginners covers the algebra foundations.