How to Find the Volume and Surface Area of a Triangular Prism?

A triangular prism has two congruent triangular bases. Its volume is base area times prism length; its surface area also includes the lateral faces.

TL;DR: Picture a Toblerone bar. That's a triangular prism, two triangular bases connected by three rectangular faces. Its volume follows the same rule every prism uses: base area times length. Surface area means adding up all five faces, both triangles plus the three rectangles wrapping around. Once you find the area of that triangular base, both formulas become quick multiplication and addition. No special shortcuts needed, just careful bookkeeping of which face is which.

Key takeaways:

Triangular prism: two triangular bases plus three rectangular side faces (5 faces total).
Volume = base area times length (length = distance between the two triangles).
Base area = (1/2)(base of triangle)(height of triangle).
Surface area = 2(area of triangle) + perimeter of triangle times length.
Total faces: 5; edges: 9; vertices: 6.

In this step-by-step guide, you learn how to use formulas to find the volume and surface area of a triangular prism.

How to Find the Volume and Surface Area of a Triangular Prism?

The prism is a solid shape with flat faces, two identical bases, and the same cross-section along its entire length. The name of a particular prism depends on the two bases of the prism, which can be triangular, rectangular, or polygonal.

Try it

Surface Area Calculator

Open the full calculator →

Type the problems from this lesson and check each step.

A step-by-step guide to finding the volume and surface area of triangular prism

A triangular prism is a three-dimensional polyhedron with three rectangular faces and two triangular faces. The \(2\) triangular faces are congruent to each other, and the \(3\) lateral faces which are in the shape of rectangles are also congruent to each other. Thus, a triangular prism has \(5\) faces, \(9\) edges, and \(6\) vertices.

See the image below of a triangular prism where \(l\) represents the length of the prism, \(h\) represents the height of the base triangle, and \(b\) represents the bottom edge of the base triangle.

Triangular prism properties

The properties of a triangular prism help us to easily identify it. The following are some features of a triangular prism:

A triangular prism has \(5\) faces, \(9\) edges, and \(6\) vertices.
It is a polyhedron with \(3\) rectangular faces and \(2\) triangular faces.
The two triangular bases are congruent with each other.
Any cross-section of a triangular prism is in the shape of a triangle.

Triangular prism formulas

There are two important formulas for a triangular prism, which are surface area and volume.

The volume of a triangular prism

The volume of a triangular prism is the product of its triangular base area and the length of the prism. As we already know that the base of a triangular prism is in the shape of a triangle. So,

\(\color{blue}{Volume\:of\:a\:triangular\:prism\:=\:area\:of\:base\:triangle\:×\:length\:=\frac{1}{2}\:×\:b\:×\:h\:×\:l}\)

where,

\(b\) is the base length of the triangle,
\(h\) is the height of the triangle,
\(l\) is the length of the prism.

The surface area of a triangular prism

The surface area of a triangular prism is the area occupied by the surface. It is the sum of the areas of all the faces of the prism. Therefore, the formula to calculate the surface area is:

\(\color{blue}{Surface\:area\:=\left(Perimeter\:of\:the\:base\:×\:Length\:of\:the\:prism\right)\:+\:\left(2\:×\:Base\:Area\right)\:=\:\left(S_1\:+S_2\:+\:S_3\right)L\:+\:bh}\)

where,

\(b\) is the lower edge of the base triangle,
\(h\) is the height of the base triangle,
\(L\) is the length of the prism,
\(S_1, S_2,\) and \(S_3\) are the three edges (sides) of the base triangle,
\((bh)\) is the combined area of the two triangular faces because \(\left[2\:×\:\left(\frac{1}{2}\:×\:bh\right)\right]\:=\:bh\).

The lateral surface area of the triangular prism

The lateral surface area of any solid is the area without the bases. In other words, the lateral surface area of a triangular prism is calculated without considering the base area. Thus, the lateral surface area of a triangular prism is:

\(\color{blue}{Lateral\:surface\:area =\:\left(S_1\:+\:S_2\:+\:S_3\right)\:×\:l\:=\:\left(Perimeter\:×\:Length\right)\:or\:LSA=\:p\:×\:l}\)

where,

\(l\) is the height (length) of a prism
\(p\) is the perimeter of the base

Geometry for Beginners The Ultimate Step by Step Guide to Acing Geometry

Finding the Volume and Surface Area of Triangular Prism, Example 1:

Find the volume of a triangular prism where the base of the triangle is \(8\:??\:\), its height is \(6\:??\:\), and the length of the prism is \(10\:??\:\).

Solution:

The base of the triangle is \((b) = 8\space in\), and the height of the triangular base \((h) = 6\space in\).

So, the base area \(= (\frac{1}{2})(bh) = (\frac{1}{2}) × (8 × 6) = \frac{48}{2}=24\space in^2\).

The length of the prism is \(L = 10\space in\).

Using the volume of the triangular prism formula,

The volume of the given triangular prism \(=base\:area\:×\:length\:of\:the\:prism = 24 × (10) = 240\space in^3\).

Exercises for Finding the Volume and Surface Area of Triangular Prism

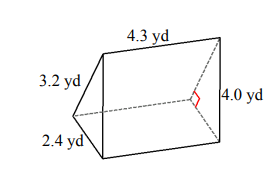
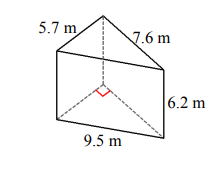
Find the volume and surface area for each triangular prism.

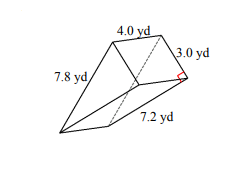
\(\color{blue}{V=43.2\:yd^3, A=93.6\:yd^2}\)
\(\color{blue}{V=16.5\:yd^3, A=49\:yd^2}\)
\(\color{blue}{V=134.3\:m^3, A=184.7\:m^2}\)

Frequently Asked Questions

How do you find the volume and surface area of a triangular prism?

Find the area of one triangular base first, using (1/2) × base × height of the triangle. Multiply that area by the prism’s length to get the volume. For surface area, add the two triangles and the three rectangles: SA = 2 × triangle area + perimeter of triangle × length. With a 3-4-5 triangle and a length of 8, the volume is 6 × 8 = 48 and the surface area is 2(6) + 12(8) = 108.

What is the formula for volume and surface area of a triangular prism?

Volume is V = (1/2)bh × L, where b and h are the base and height of the triangle and L is the length of the prism. Surface area is SA = bh + (s₁ + s₂ + s₃) × L, which is the two triangles (bh together) plus the three rectangular sides. Volume is measured in cubic units and surface area in square units, so label your answers carefully.

How do you find the surface area and volume of a right triangular prism?

A right triangular prism has a right triangle as its base, so the two legs act as the base and height of the triangle. Take legs 5 and 12 with hypotenuse 13 and a length of 10. The triangle area is (1/2)(5)(12) = 30, so V = 30 × 10 = 300 cubic units. The perimeter is 30, so SA = 2(30) + 30(10) = 360 square units.

How do you find the volume of a triangular prism?

Multiply the area of the triangular base by the length of the prism. Picture a tent-shaped box: the triangle at one end has base 6 and height 4, so its area is 12 square units. If the prism runs 10 units deep, V = 12 × 10 = 120 cubic units. A common slip is forgetting the 1/2 in the triangle area, which doubles the answer.

Can you give a triangular prism volume and surface area practice problem?

Try this one: the base is an isosceles triangle with sides 5, 5 and 8, its height is 3, and the prism is 7 units long. The triangle area is (1/2)(8)(3) = 12, so V = 12 × 7 = 84 cubic units. The perimeter is 18, so SA = 2(12) + 18(7) = 24 + 126 = 150 square units. The exercises with the lesson give more problems like this to check your skills.

Related Lessons You May Like

For a workbook on geometry, Geometry for Beginners walks every topic from first principles. Pre-Algebra for Beginners covers the algebra foundations.

Effortless Math Team

2 weeks ago

Effortless Math Team

Related to This Article

What people say about "Volume and Surface Area of a Triangular Prism"?

No one replied yet.

How to Find the Volume and Surface Area of a Triangular Prism?

Related Topics