How to Find Vertical Angles? (+FREE Worksheet!)

In this article, you will learn how to Find Vertical angles in a few simple steps.
Step by step guide to Finding Vertical angles
The intersecting lines form an \(X\)-shape, and the angles on the two opposite sides of this \(X\) are called vertical angles. The two vertical angles are always the same size and they have the same vertex. The bisector of two vertical angles makes a straight angle.
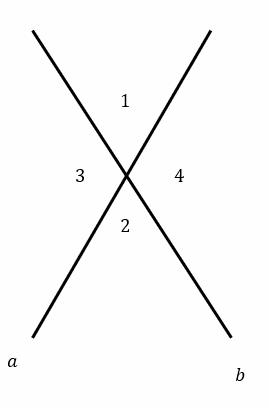
In the diagram at the right, lines and are straight:
- Angle\(1\)+ Angle\(3\)=\(180\)(because it is a straight angle)
- Angle\(2\)+ Angle\(3\)=\(180\)(because it is a straight angle)
Infer from the above two relations that angle \(1\) and \(2\) angle are equal; So, the vertical angles are equal.
In this diagram:
- Angle \(1\) and angle \(2\) are vertical angles.
- Angle \(3\) and angle \(4\) are vertical angles.
- Angle \(1\) and angle \(3\) are NOT vertical angles.
Finding Vertical Angles Example 1:
Find the number of degrees.
Solution: \((3x+50)^{\circ}\) and \((5x+10)^{\circ}\) are vertical angles.
\(3x+50=5x+10→3x+50-50=5x+10-50→3x=5x-40→3x-5x=-40→-2x=-40→x=20\)
\((3x+50)^{\circ}=(3(20)+50)=110^{\circ}\)
\((5x+10)^{\circ}=(5(20)+10=110^{\circ}\)
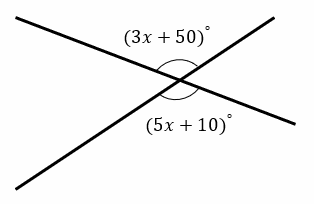
Finding Vertical Angles Example 2:
Find the number of degrees.
Solution: \((4x)^{\circ}\) and \((6x-22)^{\circ}\) are vertical angles.
\(4x=6x-22→4x-6x=-22→-2x=-22→x=11\)
\((4x)^{\circ}=(4(11))=44^{\circ}\)
\((6x-22)^{\circ}=(6(11)-22=44^{\circ}\)

Exercises for Finding Vertical Angles
Find the value of \(x\).
1)
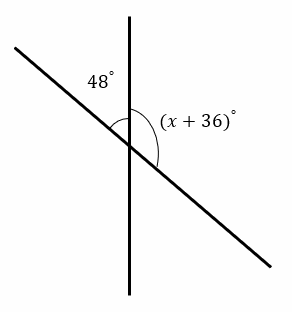
2)
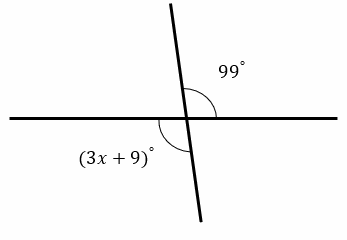

- \(x=96\)
- \(x=30\)
Related to This Article
More math articles
- 5th Grade OAA Math Worksheets: FREE & Printable
- 8th Grade NYSE Math FREE Sample Practice Questions
- The Ultimate MCAS Algebra 1 Course (+FREE Worksheets)
- FREE CBEST Math Practice Test
- 5th Grade CMAS Math Worksheets: FREE & Printable
- Product Projections: How to Swiftly Estimate Decimal Multiplications
- Intelligent Math Puzzle – Challenge 86
- List Of the Best Middle School Math Supply for Learning
- How to Graph Inequality: Using Number Lines to Graph Inequalities
- How to Choose the Best Backup Software Solution for School Districts?


























What people say about "How to Find Vertical Angles? (+FREE Worksheet!) - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.