How to Use a Venn Diagram to Classify Rational Numbers?

A Step-by-step Guide to Using a Venn Diagram to Classify Rational Numbers

To use a Venn diagram to classify rational numbers, you can follow these steps:

Step 1: Draw a large rectangle to represent the set of all real numbers.

Step 2: Draw two overlapping circles inside the rectangle, one to represent the set of rational numbers and the other to represent the set of irrational numbers.

Step 3: Label the circle representing rational numbers as \(“Q”\) and the circle representing irrational numbers as \(“I”\).

Step 4: Write down the definition of rational numbers, which are numbers that can be expressed as a ratio of two integers. This means that any number that can be written in the form of \(\frac{p}{q}\), where \(p\) and \(q\) are integers and \(q\) is not equal to zero, belongs to the set of rational numbers.

Step 5: Shade the region inside the circle \(“Q”\) to show all the rational numbers. This includes all fractions, integers, terminating decimals, and repeating decimals.

Step 6: Shade the region inside the circle \(“I”\) to show all the irrational numbers. These include numbers that cannot be expressed as a ratio of two integers, such as the square root of \(2\) or pi.

Note that the region outside both circles represents the set of real numbers that are neither rational nor irrational, such as complex numbers.

By using a Venn diagram, you can visualize the relationship between different sets of numbers and classify them accordingly.

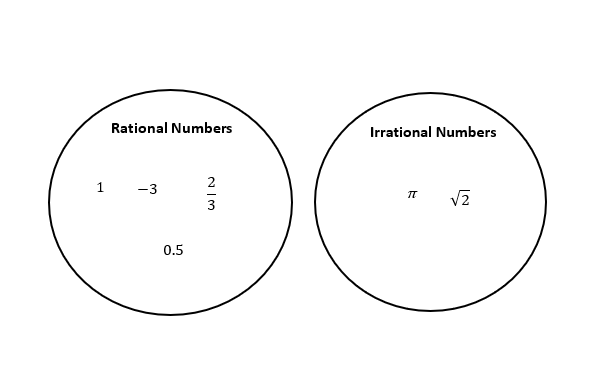
Using a Diagram to Classify Rational Numbers – Examples 1

Classify the following numbers as rational or irrational: \(1, 0.5, -3, \frac{2}{3}, \sqrt{2}, π\)

Solution:

Step 1: Draw a large rectangle to represent the set of all real numbers.

Step 2: Draw two overlapping circles inside the rectangle, one to represent the set of rational numbers and the other to represent the set of irrational numbers.

Step 3: Label the circle representing rational numbers as \(“Q”\) and the circle representing irrational numbers as \(“I”\).

Step 4: Shade the region inside the circle \(“Q”\) to show all the rational numbers. This includes all fractions, integers, terminating decimals, and repeating decimals.

Step 5: Shade the region inside the circle \(“I”\) to show all the irrational numbers. These include numbers that cannot be expressed as a ratio of two integers, such as the square root of \(2\) or pi.

Now we can classify the given numbers:

\(1\): Rational (an integer)

\(0.5\): Rational (a terminating decimal)

\(-3\): Rational (an integer)

\(\frac{2}{3}\): Rational (a fraction)

\(\sqrt{2}\): Irrational (a number that cannot be expressed as a ratio of two integers)

\(π\): Irrational (a number that cannot be expressed as a ratio of two integers)

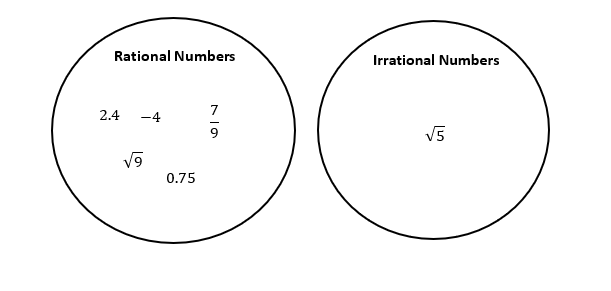
Using a Diagram to Classify Rational Numbers – Examples 2

Classify the following numbers as rational or irrational: \(-4, \frac{7}{9}, \sqrt{9}, 0.75, \sqrt{5}, 2.4\)

Solution:

Step 1: Draw a large rectangle to represent the set of all real numbers.

Step 2: Draw two overlapping circles inside the rectangle, one to represent the set of rational numbers and the other to represent the set of irrational numbers.

Step 3: Label the circle representing rational numbers as \(“Q”\) and the circle representing irrational numbers as \(“I”\).

Step 4: Shade the region inside the circle \(“Q”\) to show all the rational numbers. This includes all fractions, integers, terminating decimals, and repeating decimals.

Step 5: Shade the region inside the circle \(“I”\) to show all the irrational numbers. These include numbers that cannot be expressed as a ratio of two integers, such as the square root of \(2\) or pi.

Now we can classify the given numbers:

\(-4\): Rational (an integer)

\(\frac{7}{9}: Rational (a fraction)

\(\sqrt{9}\): Rational (an integer)

\(0.75\): Rational (a terminating decimal)

\(\sqrt{5}\): Irrational (a number that cannot be expressed as a ratio of two integers)

\(2.4\): Rational (a terminating decimal)