Top 10 8th Grade MCAS Math Practice Questions

The best way to prepare your students for the 8th Grade MCAS Math test is to help them work through as many MCAS Math practice questions as possible. Here are the top 10 8th Grade MCAS Math practice questions to help your students review the most important 8th Grade MCAS Math concepts. These 8th Grade MCAS Math practice questions are designed to cover mathematics concepts and topics that are found on the actual test. The questions have been fully updated to reflect the latest 2026 8th Grade MCAS guidelines. Answers and full explanations are provided at the end of the post.
Help your students start their MCAS Math test prep journey right now with these sample MCAS Math questions.
The Absolute Best Book to Ace 8th Grade MCAS Math Test
8th Grade MCAS Math Practice Questions
1- A rope weighs \(600\) grams per meter of length. What is the weight in kilograms of \(12.2\) meters of this rope? (\(1 \) kilograms \(= 1000\) grams)
A. \(0.0732\)
B. \(0.732\)
C. \(7.32\)
D. \(7320\)
2- In a school, the ratio of the number of boys to girls is \(3:7\). If the number of boys is \(180\), what is the total number of students in the school?__________
Write your answer in the box below.
3- In two successive years, the population of a town is increased by \(15\%\) and \(20\%\). What percent of its population is increased after two years?
A. \(32\)
B. \(35\)
C. \(38\)
D. \(68\)
4- Which graph shows a non–proportional linear relationship between \(x\) and \(y\)?
A.
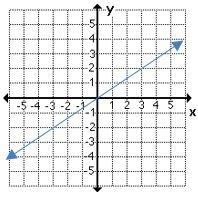
B.
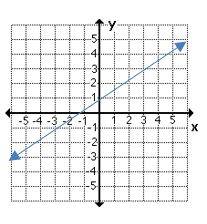
C.
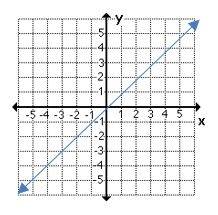
D.
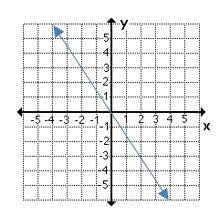
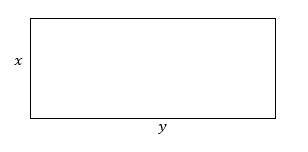
5- In the rectangle below if \(y>5\) \(cm\) and the area of a rectangle is \(50 cm^2\) and the perimeter of the rectangle is \(30 cm\), what is the value of \(x\) and \(y\) respectively?
A. \(4, 11\)
B. \(5, 11\)
C. \(5, 10\)
D. \(4, 10\)
6- A football team had \($40,000\) to spend on supplies. The team spent \($22,000\) on new balls. New sports shoes cost \($240\) each. Which of the following inequalities represents how many new shoes the team can purchase?
A. \(240x+22,000 ≤40,000 \)
B. \(240x+22,000 ≥40,000\)
C. \(22,000x+240 ≤40,000\)
D. \(22,000x+240 ≥40,000\)
7- Right triangle ABC has two legs of lengths \(6 cm\) (AB) and \(8 cm\) (AC). What is the length of the third side (BC)?
A. \(4 cm\)
B. \(6 cm\)
C. \(8 cm\)
D. \(10 cm\)
8- If \(3x-5=8.5\), What is the value of \(5x+3\)?
A. \(13\)
B. \(15.5\)
C. \(20.5\)
D. \(25.5\)
9- A bank is offering \(4.5\%\) simple interest on a savings account. If you deposit \($8,000\), how much interest will you earn in five years?
A. \($360\)
B. \($720\)
C. \($1800\)
D. \($3600\)
10- In a party, \(10\) soft drinks are required for every \(12\) guests. If there are \(252\) guests, how many soft drinks is required?
A. \(21\)
B. \(105\)
C. \(210\)
D. \(2510\)
The Absolute Best Book to Ace 8th Grade MCAS Math Test
Answers:
1- C
The weight of \(12.2\) meters of this rope is: \(12.2 × 600 g = 7320 g\)
\(1 kg = 1000 g\)
therefore,
\(7320 g ÷ 1000 = 7.32 kg\)
2- 600
The ratio of boys to girls is \(3:7\).
Therefore, there are \(3\) boys out of \(10\) students. To find the answer, first, divide the number of boys by \(3\), then multiply the result by \(10\).
\(180 ÷ 3 = 60 ⇒ 60 × 10 = 600\)
3- C
the population is increased by \(15\%\) and \(20\%\). \(15\%\) increase changes the population to \(115\%\) of the original population.
For the second increase, multiply the result by \(120\%\).
\((1.15) × (1.20) = 1.38 = 138\%\)
\(38\) percent of the population is increased after two years.
4- B
A linear equation is a relationship between two variables, \(x\) and \(y\), that can be put in the form \(y = mx + b\).
A non-proportional linear relationship takes on the form \(y=mx + b\), where \(b ≠ 0\) and its graph is a line that does not cross through the origin.
5- C
The perimeter of the rectangle is: \(2x+2y=30→x+y=15→x=15-y \)
The area of the rectangle is: \(x×y=50→(15-y)(y)=50→y^2-15y+50=0\)
Solve the quadratic equation by factoring method.
\((y-5)(y-10)=0→y=5 \)
(Unacceptable, because \(y\) must be greater than \(5\)) or \(y=10\)
If \( y=10 →x×y=50→x×10=50→x=5\)
6- A
Let \(x\) be the number of new shoes the team can purchase. Therefore, the team can purchase \(240 x\).
The team had \($40,000\) and spent \($22,000\). Now the team can spend on new shoes \($18,000\) at most.
Now, write the inequality: \( 120x+22.000 ≤40.000\)
7- D
Use Pythagorean Theorem:
\(a^2 + b^2 = c^2\)
\(6^2 + 8^2 = c^2 ⇒ 100 = c^2 ⇒ c = 10\)
8- D
\(3x-5=8.5→3x=8.5 + 5=13.5→x = \frac{13.5}{3}= 4.5\)
Then;
\(5x+3=5 (4.5)+3=22.5+3=25.5\)
9- C
Use simple interest formula:
\(I=prt\)
\((I = interest, p = principal, r = rate, t = time)\)
\(I=(8000)(0.045)(5)=1800\)
10- C
Let x be the number of soft drinks for \(252\) guests. Write the proportion and solve for \(x\).
\(\frac{10 soft drinks}{12 guests}=\frac{x}{252 guests}\)
\(x = \frac{252×10}{12}⇒x=210\)
Looking for the best resource to help you succeed on the 8th Grade MCAS Math test?
The Best Books to Ace 8th Grade MCAS Math Test
Common Core Math Exercise Book for Grade 8 Student Workbook and Two Realistic Common Core Math Tests
Related to This Article
More math articles
- 7th Grade Wisconsin Forward Math Worksheets: FREE & Printable
- Number Properties Puzzle – Challenge 4
- 10 Most Common 3rd Grade OST Math Questions
- The Best Strategies For Successful Math Tutoring Online
- How Math Can Help You Win At Poker?
- Quadratic Function
- Top 10 8th Grade MAP Math Practice Questions
- Can Dice Models Compete with Lightning-Fast Layer 2 Systems?
- PSAT 10 Math Practice Test Questions
- Tips on Hiring a Great Math tutor


















What people say about "Top 10 8th Grade MCAS Math Practice Questions - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.