The Ultimate ATI TEAS 7 Math Formula Cheat Sheet

Mixed Numbers

A number is composed of a whole number and a fraction. Example: \(2 \frac{2}{ 3}\) Converting between improper fractions and mixed numbers: \(a \frac{c}{b}=a+\frac{c}{b}= \frac{ab+ c}{b}\)

Factoring Numbers

Factor a number means breaking it up into numbers that can be multiplied together to get the original number. Example:\(12=2×2×3\)

Integers  

\( \{…,-3,-2,-1,0,1,2,3,…\} \)
Includes: zero, counting numbers, and the negative of the counting numbers

Real Numbers  

All numbers that are on a number line. Integers plus fractions, decimals, and irrationals, etc.) (\(\sqrt{2},\sqrt{3},π\), etc.)

Order of Operations  

PEMDAS
(parentheses/ exponents/ multiply/ divide/ add/ subtract)

Absolute Value

Refers to the distance of a number from \(0\) on the number line. the distances are positive as the absolute value of a number cannot be negative. \(|-22|=22\)
or \(|x| =\begin{cases}x \ for \ x≥0 \\x \ for \ x < 0\end{cases} \)
\(|x|<n⇒-n<x<n\)
\(|x|>n⇒x<-n or x>n\)

Ratios

A ratio is a comparison of two numbers by division.
Example: \(3: 5\), or \(\frac{3}{5}\)

Percentages

Use the following formula to find part, whole, or percent
part \(=\frac{percent}{100}×whole\)

Proportional Ratios

A proportion means that two ratios are equal. It can be written in two ways:  
\(\frac{a}{b}=\frac{c}{d}\), \(a: b = c: d  \)

Percent of Change

\(\frac{New \ Value \ – \ Old \ Value}{Old Value}×100\%\)

Expressions and Variables  

A variable is a letter that represents unspecified numbers. One may use a variable in the same manner as all other numbers: Addition: \(2+a\): \(2\) plus a
Subtraction: \(y-3\)  : \(y\) minus \(3\)
Division: \(\frac{4}{x}\)  : 4 divided by x
Multiplication: \(5a\)  : \(5\) times a

Distributive Property  

\(a(b+c)=ab+ac\)

Equations  

The values of the two mathematical expressions are equal.
\(ax+b=c\)

Distance from A to B:

\(\sqrt{(x_{1}-x_{2})^2+(y_{1}-y_{2})^2 }\)

Parallel and Perpendicular lines:  

Have equal slopes. Perpendicular lines (i.e., those that make a \(90^° \) angle where they intersect) have negative reciprocal slopes: \(m_{1}\).\(m_{2}=-1\).
Parallel Lines (l \(\parallel\) m)

Mid-point of the segment AB:  

M (\(\frac{x_{1}+x_{2}}{2}, \frac{y_{1}+y_{2}}{2}\))

Slope of the line:  

\(\frac{y_{2}- y_{1}}{x_{2} – x_{1} }=\frac{rise}{run}\)

Point-slope form:  

Given the slope m and a point \((x_{1},y_{1})\) on the line, the equation of the line is
\((y-y_{1})=m \ (x-x_{1})\).

Slope-intercept form:

given the slope m and the y-intercept b, then the equation of the line is:
\(y=mx+b\).

Factoring:

“FOIL”
\((x+a)(x+b)\)
\(=x^2+(b+a)x +ab\) “Difference of Squares”
\(a^2-b^2= (a+b)(a-b)\)
\(a^2+2ab+b^2=(a+b)(a+b) \)
\(a^2-2ab+b^2=(a-b)(a-b)\) “Reverse FOIL”
\(x^2+(b+a)x+ab=\) \((x+a)(x+b)\)

You can use Reverse FOIL to factor a polynomial by thinking about two numbers a and b which add to the number in front of the x, and which multiply to give the constant. For example, to factor \(x^2+5x+6\), the numbers add to 5 and multiply to 6, i.e.: \(a=2\) and \(b=3\), so that \(x^2+5x+6=(x+2)(x+3)\). To solve a quadratic such as \(x^2+bx+c=0\), first factor the left side to get \((x+a)(x+b)=0\), then set each part in parentheses equal to zero. For example, \(x^2+4x+3= (x+3)(x+1)=0\) so that \(x=-3\) or \(x=-1\).
To solve two linear equations in x and y: use the first equation to substitute for a variable in the second. E.g., suppose \(x+y=3\) and \(4x-y=2\). The first equation gives y=3-x, so the second equation becomes \(4x-(3-x)=2 ⇒ 5x-3=2\) \(⇒ x=1,y=2\).

Exponents:  

Refers to the number of times a number is multiplied by itself.
\(8 = 2 × 2 × 2 = 2^3\)

Scientific Notation:  

It is a way of expressing numbers that are too big or too small to be conveniently written in decimal form.
In scientific notation all numbers are written in this form: \(m \times 10^n\)
Decimal notation:
5
\(-25,000\)
0.5
2,122.456
Scientific notation:
\(5×10^0\)
\(-2.5×10^4\)
\(5×10^{-1}\)
\(2,122456×10^3\)

Square:  

The number we get after multiplying an integer (not a fraction) by itself. Example: \(2×2=4,2^2=4\)

Square Roots:

A square root of \(x\) is a number r whose square is \(x: r^2=x\)
\(r\) is a square root of \(x\)

Pythagorean Theorem:  

For any right triangle with legs \(a\) and \(b\) and hypotenuse \(c\): \(a^2+b^2=c^2\)
Solving for the hypotenuse: \(c=\sqrt{a^2+b^2}\)
Solving for a leg: \(a=\sqrt{c^2-b^2}\)
Common Pythagorean triples: \(3,4,5\); \(5,12,13\); \(8,15,17\); \(7,24,25\)

Triangles

Area: \(A=\frac{1}{2}bh\) where \(b\) is the base and \(h\) is the height.
Perimeter: \(P=a+b+c\) (sum of all three sides).
Pythagorean Theorem (right triangles): \(a^2+b^2=c^2\) where \(c\) is the hypotenuse.
Sum of interior angles: \(180°\)

All triangles:

Area \(=\frac{1}{2}\) b. h
Angles on the inside of any triangle add up to \(180^\circ\).
The length of one side of any triangle is always less than the sum and more than the difference between the lengths of the other two sides.
An exterior angle of any triangle is equal to the sum of the two remote interior angles. Other important triangles:

Equilateral:  

These triangles have three equal sides, and all three angles are \(60^\circ\).

Isosceles:

An isosceles triangle has two equal sides. The “base” angles (the ones opposite the two sides) are equal (see the \(45^\circ\)  triangle above).

Circles

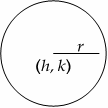
Area \(=πr^2\)
Circumference \(=2πr\)
Full circle \(=360^\circ\)

Rectangles

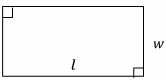
(Square if l=w)
Area=lw

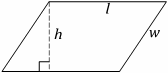
Parallelogram

(Rhombus if l=w)
Area=lh
Regular polygons are n-sided figures with all sides equal and all angles equal.
The sum of the inside angles of an n-sided regular polygon is
\((n-2).180^\circ\).

Area of a trapezoid:  

\(A =\frac{1}{2} h (b_{1}+b_{2})\)

Surface Area and Volume of a Rectangular/right prism:  

\(SA=ph+2B\)
\(V=Bh\)

Surface Area and Volume of a Cylinder:

\(SA =2πrh+2πr^2\)
\(V =πr^2 h  \)

Surface Area and Volume of a Cone  

\(SA =πrs+πr^2\)
\(V=\frac{1}{3} \ πr^2 \ h\)

Surface Area and Volume of a Sphere  

\(SA =4πr^2\)
\(V =\frac{4}{3} \ πr^3\)
(p \(=\) perimeter of base B; \(π ~ 3.14 \))

The Ultimate Algebra Bundle 2026 From Pre-Algebra to Algebra II

Download

$109.99 Original price was: $109.99.$54.99Current price is: $54.99.

Simple interest:

\(I=prt\)
(I = interest, p = principal, r = rate, t = time)

mean:

mean: \(\frac{sum \ of \ the \ data}{of \ data \ entires}\)

mode:

value in the list that appears most often

range:

largest value \(-\) smallest value

Median  

The middle value in the list (which must be sorted)
Example: median of
\( \{3,10,9,27,50\} = 10\)
Example: median of
\( \{3,9,10,27\}=\frac{(9+10)}{2}=9.5 \)

Average

\( \frac{sum \ of \ terms}{number \ of \ terms}\)

Average speed

\(\frac{total \ distance}{total \ time}\)

Probability

\(\frac{number \ of \ desired \ outcomes}{number \ of \ total \ outcomes}\)
The probability of two different events A and B both happening is:
P(A and B)=p(A).p(B)
as long as the events are independent (not mutually exclusive).

Powers, Exponents, Roots

\(x^a.x^b=x^{a+b}\)
\(\frac{x^a}{x^b} = x^{a-b}\)
\(\frac{1}{x^b }= x^{-b}\)
\((x^a)^b=x^{a.b}\)
\((xy)^a= x^a.y^a\)
\(x^0=1\)
\(\sqrt{xy}=\sqrt{x}.\sqrt{y}\)
\((-1)^n=-1\), if n is odd.
\((-1)^n=+1\), if n is even.
If \(0<x<1\), then
\(0<x^3<x^2<x<\sqrt{x}<\sqrt{3x}<1\).

Simple Interest

The charge for borrowing money or the return for lending it.
Interest = principal \(×\) rate \(×\) time
OR
\(I=prt\)

Powers/ Exponents

\(x^a×x^b=x^{a+b}\)
\(\frac{x^a}{x^b}=x^{a-b}\)
\((x^a)^b=x^{ab}\)
\(x^0=1\)
\(x^{-a}=\frac{1}{x^a}\)
\(x^{\frac{1}{n}}=\sqrt[n]{x}\)

Positive Exponents

An exponent is simply shorthand for multiplying that number of identical factors. So \(4^3\) is the same as (4)(4)(4), three identical factors of 4. And \(x^3\) is just three factors of \(x\), \((x)(x)(x)\).

Negative Exponents

A negative exponent means to divide by that number of factors instead of multiplying.
So \(4^{-3}\) is the same as \( \frac{1}{4^3}\) and
\(x^{-3}=\frac{1}{x^3}\)

Factorials  

Factorial- the product of a number and all counting numbers below it.
8 factorial \(=8!=\)
\(8×7×6×5×4×3×2×1=40,320\)
5 factorial \(=5!=\)
\(5×4×3×2×1=120\)
2 factorial \(=2!=2× 1=2\)

Multiplying Two Powers of the SAME Base  

When the bases are the same, you find the new power by just adding the exponents
\(x^a.x^b=x^{a+b }\)

Powers of Powers

For the power of power: you multiply the exponents.
\((x^a)^b=x^{(ab)}\)

Dividing Powers

\(\frac{x^a}{x^b} =x^a x^{-b}= x^{a-b}\)

The Zero Exponent

Anything to the 0 power is 1.
\(x^0= 1\)