How to Solve Pythagorean Theorem Problems? (+FREE Worksheet!)
In mathematics, the Pythagorean Theorem is the relationship between three sides of a right triangle.
Related Topics
- How Calculate the Area of Trapezoids
- How to Find the volume and surface area of Rectangular Prisms
- How to Solve Triangles Problems
- How to Find Volume and Surface Area of Cubes
- How to Find the Perimeter of Polygons
Step by step guide to solve Pythagorean Theorem problems
- We can use the Pythagorean Theorem to find a missing side in a right triangle.
- In any right triangle: \(\color{blue}{a^2+b^2= c^2}\)
The Absolute Best Books to Ace Pre-Algebra to Algebra II
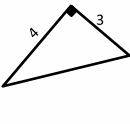
The Pythagorean Theorem – Example 1:
Right triangle ABC has two legs of lengths \(9\) cm (AB) and \(12\) cm (AC). What is the length of the third side (BC)?
Solution:
Use Pythagorean Theorem: \(\color{blue}{a^2+b^2= c^2}\)
Then: \(a^2+b^2= c^2 →9^2+12^2= c^2 →81+144=c^2\)
\(c^2=225 →\) \(c=\sqrt{225}=15\) \(cm\) → \(c=15 cm\)
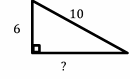
The Pythagorean Theorem – Example 2:
Find the hypotenuse of the following right triangle. 
Solution:
Use Pythagorean Theorem: \(\color{blue}{a^2+b^2= c^2}\)
Then: \(a^2+b^2= c^2 →8^2+6^2= c^2 →64+36=c^2\)
\(c^2=100 →\) \(c=\sqrt{100}=10\) → \(c=10\)
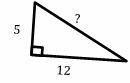
The Pythagorean Theorem – Example 3:
Find the hypotenuse of the following right triangle.
Solution:
Use Pythagorean Theorem: \(\color{blue}{a^2+b^2= c^2}\)
Then: \(a^2+b^2= c^2 →3^2+4^2= c^2 →9+16=c^2\)
\(c^2=25 →\) \(c=\sqrt{25}=5\) → \(c=5\)
The Best Book to Help You Ace Pre-Algebra
The Pythagorean Theorem – Example 4:
Right triangle ABC has two legs of lengths \(6\) cm (AB) and \(8\) cm (AC). What is the length of the third side (BC)?
Solution:
Use Pythagorean Theorem: \(\color{blue}{a^2+b^2= c^2}\)
Then: \(a^2+b^2= c^2 →6^2+8^2= c^2 →36+64=c^2\)
\( c^2=100 →\) \(c=\sqrt{100}=10\) \(cm\) → \(c= 10 cm\)
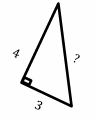
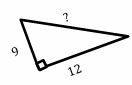
Exercises for Solveing the Pythagorean Theorem
Find the missing side in each right triangle.
Download Pythagorean Relationship Worksheet
- \(\color{blue}{13}\)
- \(\color{blue}{5}\)
- \(\color{blue}{15}\)
- \(\color{blue}{8}\)
The Greatest Books for Students to Ace the Algebra
Related to This Article
More math articles
- FREE 6th Grade FSA Math Practice Test
- Full-Length SSAT Middle Level Practice Test-Answers and Explanations
- How to Apply Integers Addition and Subtraction Rules?
- Real-Life Math Problem With Credit Card Utilization
- Top 10 Tips You MUST Know to Retake the AFOQT Math
- Finding Area of Compound Figures
- The Best AFOQT Math Worksheets: FREE & Printable
- 5th Grade RISE Math Worksheets: FREE & Printable
- 6th Grade LEAP Math Worksheets: FREE & Printable
- How to Evaluate Trigonometric Function? (+FREE Worksheet!)
What people say about "How to Solve Pythagorean Theorem Problems? (+FREE Worksheet!) - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.