Special Right Triangles
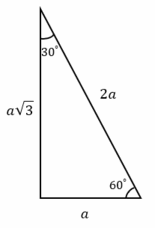
Special Right Triangles, Example 2:
Exercises for Special Right Triangles
Find the value of x and y in each triangle.
1-
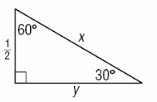
2-
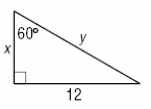
3-
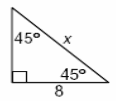
4-
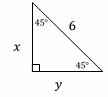
5-
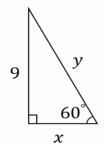
6-
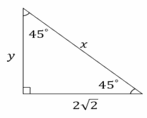
- (color{blue}{x=1,y=frac{sqrt{3}}{2}})
- (color{blue}{x=4sqrt{3},y=8sqrt{3}})
- (color{blue}{x=8sqrt{2}})
- (color{blue}{x=3sqrt{2},y=3sqrt{2}})
- (color{blue}{x=3sqrt{3},y=6sqrt{3}})
- (color{blue}{x=4,y=2sqrt{2}})
The Absolute Best Book for the Algebra Test
Original price was: $29.99.$19.99Current price is: $19.99.
Original price was: $109.99.$54.99Current price is: $54.99.
Related to This Article
More math articles
- Full-Length HiSET Math Practice Test
- Counting the Change: How to Master the Subtraction of Money Amounts
- How to Divide by Zero?
- The Best Grade 4 Math Book for Pennsylvania Students
- Accuplacer Math Formulas
- 5th Grade MCAS Math Practice Test Questions
- Tenements, Streetcar Suburbs, and Political Machines
- South Dakota Algebra 1 Free Worksheets: Printable Standards-Aligned Algebra 1 PDFs, Free
- What Kind of Math Is on the HiSET Test?
- How Political Events Shape Ideology
What people say about "Special Right Triangles Explained"?
No one replied yet.