Slope Fields Simplified: Understanding the Core of Differential Equations

The concept of slope fields, originating in the \(18^th\) century, is attributed to Isaac Newton and Gottfried Wilhelm Leibniz, founders of calculus. Their work laid the groundwork for visualizing differential equations, with slope fields evolving as a critical tool for understanding these equations’ graphical solutions.

How to use slope fields to find the answer to a differential equation:

Understand the Differential Equation:

You should have a first-order differential equation in the form of \(\frac{dy}{dx} = f(x, y)\), where \( y \) is the dependent variable, \( x \) is the independent variable, and \( f(x, y) \) is a function involving both variables.

Determine the Region of Interest:

Identify the region of the \(xy-plane\) where you want to find solutions or where the problem is defined. It’s essential to choose a region that contains the initial conditions (if given) or the range of interest.

Choose Grid Points:

Divide the region of interest into a grid of points. These points will serve as the starting points for drawing line segments that represent the slope of the solution at each point. The grid should be fine enough to capture the behavior of the solutions accurately.

Calculate Slopes:

For each grid point \( (x, y) \), calculate the slope at that point using the differential equation’s right-hand side, \( f(x, y) \). The slope at a particular point is given by the value of \( f(x, y) \) at that point.

Draw Line Segments:

At each grid point, draw a short line segment with a slope equal to the calculated value of \( f(x, y) \). These line segments indicate the direction in which the solution curve would travel at that point.

Connect Line Segments:

Connect the line segments smoothly to form a continuous curve. This curve represents an approximate solution trajectory for the differential equation. You can use the slope field to sketch multiple solution trajectories if needed.

Analyze the Solutions:

Examine the slope field to identify the behavior of the solutions. Look for equilibrium points (where the slopes are zero) and how solutions approach or diverge from these points. You can also identify trends, such as where the solutions are increasing or decreasing.

Incorporate Initial Conditions:

If you have initial conditions (e.g., \( y(x0) = y0 \)), you can use the slope field to estimate the behavior of the solution that passes through that point. Follow the direction of the slope field to trace the solution curve from the initial condition.

Refine and Repeat:

If necessary, refine your slope field by adding more grid points or extending the region of interest to improve the accuracy of your approximate solutions.

Analyze Numerically:

For more precise solutions, you may need to use numerical methods (e.g., Euler’s method, Runge-Kutta methods) to find specific values of the solution at desired points.

Example:

Consider the first-order differential equation:

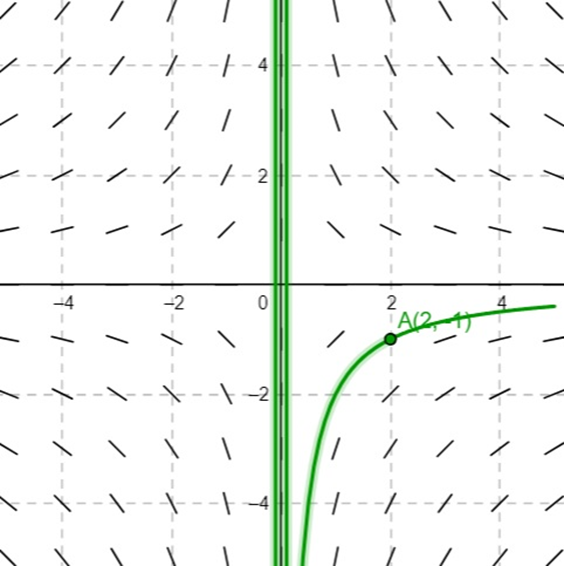
\(\frac{dy}{dx} = -\frac{y}{x}\)

1. Create a slope field for this differential equation in the region \(1 \leq x \leq 5\) and \(1 \leq y \leq 5\).
2. Use the slope field to sketch the approximate solution curve that passes through the point \((2, 2)\).
3. Determine the behavior of the solution as \(x\) approaches \(0\).

Solution:

To create the slope field, follow these steps:

1. Determine the region of interest in the \((x, y)\) plane.
2. Choose a grid of points in this region.
3. Calculate the slopes at each grid point using the differential equation.
4. Draw short line segments at each point to represent the slopes.
5. Connect these line segments to visualize the solution trajectories.

Using the slope field, sketch the approximate solution curve that passes through the point \((2, 2)\).

Behavior as \(x\) approaches \(0\):

Analyze the behavior of the solution as \(x\) approaches \(0\) based on your slope field and solution curve.

Frequently Asked Questions

What is a slope field?

A visual representation of a first-order differential equation. At sample points across the plane, you draw short line segments whose slopes equal dy/dx at that point. Solution curves follow the segments.

Why are slope fields useful?

They show the qualitative shape of solutions without solving the equation algebraically. For equations that have no closed-form solution, slope fields are often the best visualization.

How do I draw a slope field by hand?

Pick a grid of (x, y) points. At each point, compute dy/dx = f(x, y), and draw a short segment with that slope. Repeat across the grid until you see the pattern.

How do I follow a solution curve?

Pick a starting point. Move in the direction the slope segment points. After a tiny step, recompute the slope from the new point and continue. The path you trace is a solution.

What does dy/dx = y look like?

Segments have slope equal to the y-coordinate. Solutions grow exponentially (e^x family). Above the x-axis, the slopes are positive and steeper as y grows.

What does dy/dx = x look like?

Segments have slope equal to the x-coordinate. Solutions are parabolas — y = (x^2)/2 + C. To the right of the y-axis, slopes are positive and grow with x.

How do equilibrium solutions show up?

As horizontal lines where dy/dx = 0. In the slope field, these are levels where all the segments are horizontal. Solutions tend toward or away from these lines depending on the sign of the slope nearby.

Can a slope field have multiple solutions through the same point?

Generally no — the existence and uniqueness theorem says that under mild smoothness conditions, exactly one solution passes through each point. Slope fields visualize this neatly: through each point there is one curve.

Where do slope fields appear in courses?

AP Calculus AB and BC, introductory differential equations courses, and many physics modeling courses. They are also a staple of numerical analysis (Euler’s method visualizes a slope field with a step-following solution).

What tools plot slope fields?

Desmos, GeoGebra, Wolfram Alpha, MATLAB, Python (matplotlib), and many graphing calculators (TI-Nspire CAS) all have slope field plotters. Hand-sketching a small grid is also a great exercise.

Related Lessons You May Like

• How to find the slope of a line
• How to find the equation of a line
• How to find the derivative of a function
• How to solve systems of equations
• How to find volume by spinning: disk method

For a friendly introduction to slope fields and differential equations, Calculus for Beginners walks through slope fields, separable equations, and basic integration. Pre-Calculus for Beginners covers the algebra and trig prerequisites.