Limits: What Happens When a Function Approaches Infinity

A limit at infinity describes the value a function settles toward as x grows without bound, which is what determines a horizontal asymptote. Below: the definition, how to evaluate limits of rational, exponential and radical functions at infinity, and worked examples of each.

What is a Limit?

Before limits at infinity, it’s important to understand what a limit is. In simple terms, a limit examines what happens to a function \( f(x) \) as \( x \) approaches a certain value. Mathematically, this is denoted as:

\(lim_{{x \to a}} f(x) = L\)

This means that as \( x \) gets closer and closer to \( a \), the function \( f(x) \) gets closer and closer to the value \( L \).

Limits at Infinity

Limits at infinity are an extension of this concept. Instead of looking at what happens as \( x \) approaches a finite number, we explore what happens as \( x \) goes towards infinity \( \infty \) or negative infinity \( -\infty \).

This is expressed as:

\(lim_{{x \to \infty}} f(x) = L\)

or

\(lim_{{x \to -\infty}} f(x) = M\)

Here, \( L \) and \( M \) are the values that the function approaches as \( x \) becomes very large or very small, respectively.

Why are Limits at Infinity Important?

Limits at infinity are important because they give us a way to understand the end behavior of functions. This is fundamental in many areas of calculus, such as in studying asymptotes, evaluating improper integrals, and understanding the behavior of infinite series.

Examples

1. Polynomial Functions: Consider the function \( f(x) = x^2 \). As \( x \) approaches infinity, \( f(x) \) also approaches infinity. So, we write: \(lim_{{x \to \infty}} x^2 = \infty\)
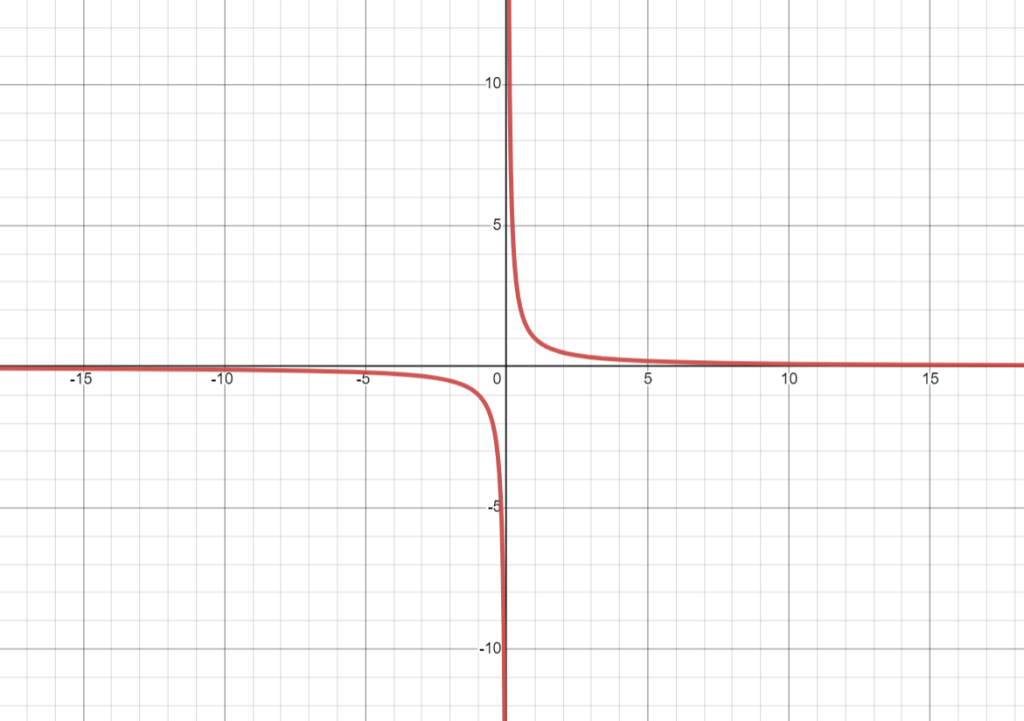
2. Rational Functions: For \( f(x) = \frac{1}{x} \), as \( x \) approaches infinity, \( f(x) \) approaches \(0\). Therefore: \(\lim_{{x \to \infty}} \frac{1}{x} = 0\)

Conclusion

Understanding limits at infinity is a fundamental part of calculus and provides deep insights into the behavior of functions. Whether it’s a polynomial, rational, or any other type of function, analyzing its behavior as inputs become very large or very small is necessary for a thorough understanding of its overall characteristics.

FAQ

What exactly does it mean when we say a limit approaches infinity?

When we say a limit approaches infinity, we mean that as the input to the function (usually denoted as \( x \)) becomes larger and larger, the value of the function grows without bound. It’s a way of describing the behavior of the function at extreme values of \( x \).

Can a limit at infinity be a finite number?

Yes, a limit at infinity can be a finite number. This happens when, despite the input growing indefinitely, the function approaches a specific, constant value. For example, \( \lim_{{x \to \infty}} \frac{1}{x} = 0 \).

How do limits at infinity apply to real-world situations?

Limits at infinity can model situations that involve large-scale behavior, such as the long-term behavior of a population in biology or the behavior of an investment over a very long time in finance.

What is the difference between a limit at infinity and an infinite limit?

A limit at infinity refers to the behavior of a function as the input goes to infinity. An infinite limit means that the function itself grows without bound as the input approaches a certain finite value. For instance, as \( x \) approaches \(0\), \( \frac{1}{x} \) becomes infinitely large.

Are limits at infinity always easy to determine?

Not always. While some functions have straightforward limits at infinity, others, especially those involving more complex expressions, may require detailed analysis or advanced calculus techniques to determine their behavior at infinity.

Does every function have a limit at infinity?

No, not every function has a well-defined limit at infinity. For some functions, the behavior as \( x \) approaches infinity may not settle towards a particular value or may oscillate indefinitely.

How does understanding limits at infinity help in calculus?

Knowing limits at infinity is essential for understanding the end behavior of functions, which is essential in areas like determining asymptotic behavior, solving improper integrals, and analyzing infinite series.