How to Write a Linear Inequality from a Graph?

A Step-by-step Guide to Write a Linear Inequality from a Graph

Here are the steps to write a linear inequality from a graph:

Step 1: Identify the slope and \(y\)-intercept of the line

The slope is the steepness of the line, and the \(y\)-intercept is the point where the line crosses the \(y\)-axis.

Step 2: Determine whether the line is solid or dashed

If the line is solid, this means that the points on the line are included in the inequality. If the line is dashed, this means that the points on the line are not included in the inequality. If the line is solid, include the equal sign in the inequality. If the line is dashed, exclude the equal sign.

Step 3: Determine whether the inequality is “greater than” or “less than

If the line slopes upwards from left to right, the inequality is “greater than.” If the line slopes downwards from left to right, the inequality is “less than.”

Step 4: Write the inequality using \(“y”\) for the \(y\)-coordinate and \(“x”\) for the \(x\)-coordinate

If the line is “greater than,” use the “greater than” symbol \((>)\); if the line is “less than,” use the “less than” symbol \((<)\). Use the slope and \(y\)-intercept to write the inequality in slope-intercept form: \(y = mx + b\), where \(“m”\) is the slope and \(“b”\) is the \(y\)-intercept.

Step 5: Write the final inequality

Write the final inequality, using the symbols for “greater than” or “less than” and the values of \(“m”\) and \(“b”\) from the equation.

Writing a Linear Inequality from a Graph – Examples 1

Write the slope-intercept form equation of the following graph.

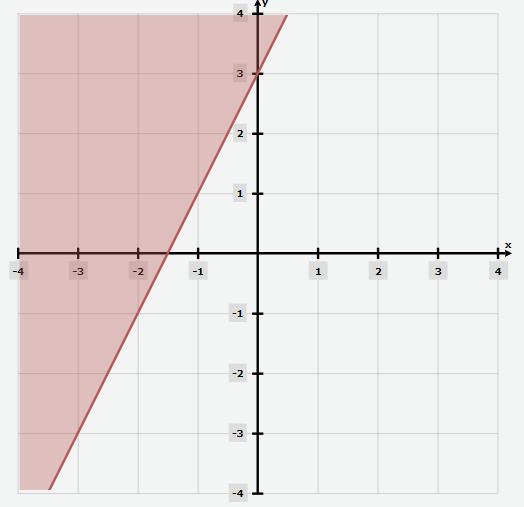
Solution:

To write the inequality equation in slope-intercept form, you should find the \(y\)-intercept \((b)\), and the slope \((m)\), of the solid line. The value of \(b\) is \(3\) because the solid line passes through the \(y\)-axis at \((0,3)\).

Now consider \(2\) points on the solid line to find the slope. You can use \((0,3)\) and \((-2,-1)\):

\(m=\frac{(y_2 – y_1)}{(x_2 – x_1)}=\frac{-1-3}{-2-0}=\frac{-4}{-2}=2→m=2\).

Now use the value of \(b\) and \(m\) and put them into the slope-intercept form formula: \(y=mx+b→y=2x+3\).

Determine the symbol of inequality: you have a solid line and the shaded part is above the line. So, the equation of the inequality is as follows: \(y≥2x+3\).

Exercises for Writing a Linear Inequality from a Graph

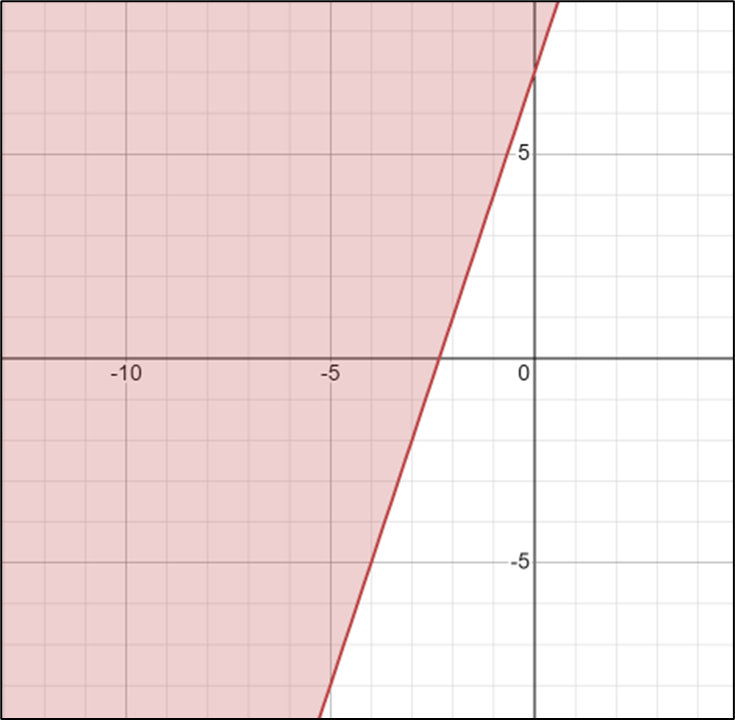
Write the slope-intercept form equation of the following graph.

1.

2.

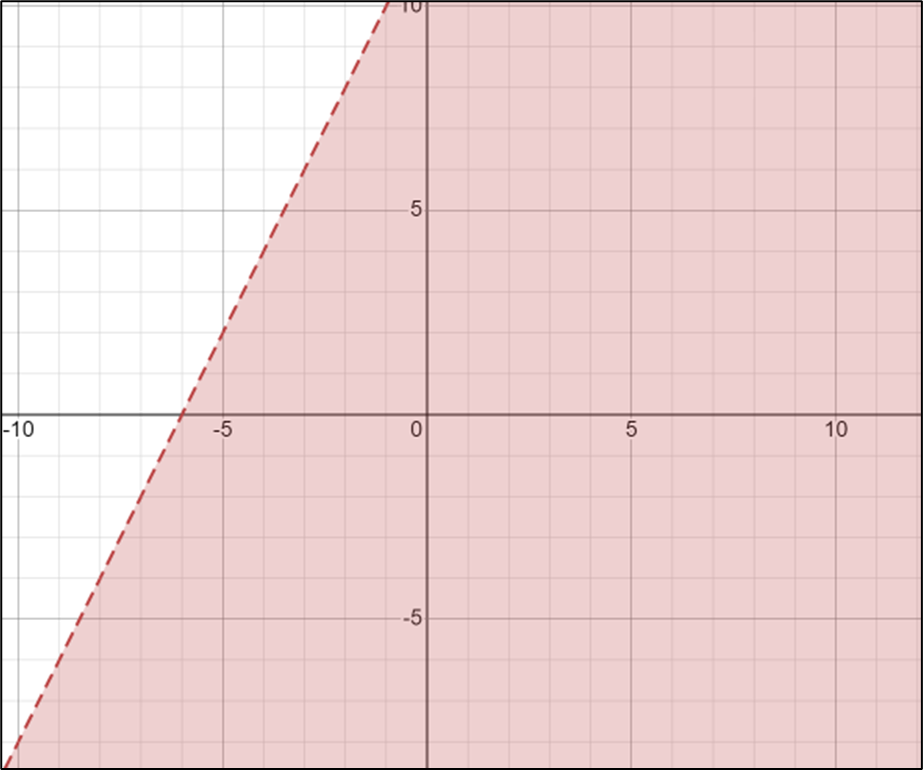
1. \(\color{blue}{y≥3x+7}\)
2. \(\color{blue}{y<2x+12}\)