How to Use Number Lines for Multiplication by a Negative Integer?
- Count the number of negative signs.
- If the number of negative signs is even, then the result is positive.
- If the number of negative signs is odd, then the result is negative.
Using Number Lines for Multiplication by a Negative Integer – Examples 1
Solution:
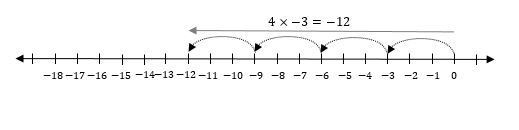
Using Number Lines for Multiplication by a Negative Integer – Examples 2
Use this formula to find the sign of the product:
(negative)\(×\)(positive)\(=\)negative
To graph \(4×-3\):
Step 1: Start from \(0\).
Step 2: Jump \(3\) units to the left side \(4\) times.
Step 3: The last jump ends at \(-12\).
Original price was: $109.99.$54.99Current price is: $54.99.
Original price was: $109.99.$54.99Current price is: $54.99.
Original price was: $109.99.$54.99Current price is: $54.99.
Related to This Article
More math articles
- Best Cheap Laptops for College Students
- Grade 5 Literary Analysis: Theme, Character Change, and Text Evidence Made Practical
- 4th Grade MCAS Math Practice Test Questions
- ISEE Middle-Level Math FREE Sample Practice Questions
- How to Find Fractions of Time Units
- How to Discover the Solutions: “HSPT Math for Beginners” Comprehensive Guide
- The Ultimate 7th Grade NYSTP Math Course (+FREE Worksheets)
- 7th Grade Georgia Milestones Assessment System Math Worksheets: FREE & Printable
- Understanding the Integral Test for Series Convergence
- Lines of Symmetry for 4th Grade
What people say about "How to Use Number Lines for Multiplication by a Negative Integer? - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.