How to Use Models to Multiply Two Fractions?

A step-by-step guide to using models to multiply two fractions

Here’s a step-by-step guide to using models to multiply two fractions:

Step 1: Identify the two fractions to be multiplied

Identify the two fractions that need to be multiplied. For example, if you need to multiply \(\frac{2}{3}\) and \(\frac{3}{4}\), these are the two fractions you will be using.

Step 2: Choose a model

Choose a model to represent the fractions. Some common models include number lines, area models, and rectangular grids. For example, you might choose an area model to represent the fractions.

To find the product of the multiplications, you can use the area model:

– The denominator in the first factor signifies the number of columns that you must draw.
– The numerator signifies the number of columns that you must shade.
– The denominator in the second factor signifies the number of rows that you must draw.
– The numerator signifies the number of rows that you must shade.

Step 3: Draw the model

Draw the model to represent the two fractions. For example, if you are using an area model, draw two rectangles, one to represent each fraction. The size of each rectangle should be proportional to the value of the fraction.

Step 4: Divide the rectangles

Divide each rectangle into the appropriate number of parts to represent the denominator of each fraction. For example, if the first rectangle represents \(\frac{2}{3}\), divide it into three equal parts. If the second rectangle represents \(\frac{3}{4}\), divide it into four equal parts.

Step 5: Shade the appropriate parts

Shade the appropriate parts of each rectangle to represent the numerator of each fraction. For example, if the first fraction is \(\frac{2}{3}\), shade two out of the three parts of the first rectangle. If the second fraction is \(\frac{3}{4}\), shade three out of the four parts of the second rectangle.

Pre-Algebra for Beginners 2026 The Ultimate Step by Step Guide to Preparing for the Pre-Algebra Test

Download

$27.99 Original price was: $27.99.$17.99Current price is: $17.99.

Rated 4.30 out of 5 based on 105 customer ratings

Satisfied 92 Students

Step 6: Count the shaded parts

Count the total number of shaded parts in the model. For example, in the area model, you would count the total number of shaded squares.

Step 7: Simplify the result

Simplify the result to its lowest terms, if necessary. For example, if the total number of shaded parts is \(6\) out of \(12\), you would simplify this to \(\frac{1}{2}\).

Using Models to Multiply Two Fractions – Example 1

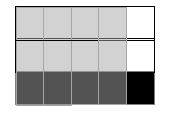
Find the product by model.
\(\frac{4}{5}×\frac{1}{3}=\)_?
The model has \(5\) columns and has \(3\) rows.
\(4\) out of \(5\) columns are shaded, which shows a factor \(\frac{4}{5}\).
\(1\) out of \(3\) rows is shaded, which shows the factor \(\frac{1}{3}\).
The part where the shaded columns and rows overlap signifies the product. There are \(4\) overlapped sections.
So, \(\frac{4}{5}×\frac{1}{3}=\frac{4}{15}\)

Recommended EffortlessMath Books

For a workbook that builds area-model fraction work into a complete operations toolkit, the Grade 5 Math for Beginners walks through fraction multiplication with models and the standard rule side by side. For broader pre-algebra coverage, the Pre-Algebra for Beginners connects fraction skills to the rest of the curriculum.

Frequently Asked Questions

What is using models to multiply two fractions?

It’s a visual method where you draw a rectangle (the unit square), shade rows for one fraction, shade columns for the other, then count overlapping cells. The overlap is the product’s numerator; the total cells are the denominator. The drawing matches the “multiply across” rule exactly.

How do you multiply two fractions with a model step by step?

Draw a rectangle. Split into horizontal rows using the first fraction’s denominator. Shade rows equal to the first numerator. Split the same rectangle into vertical columns using the second fraction’s denominator. Shade columns equal to the second numerator. Count overlap cells (numerator) and total cells (denominator). Simplify.

What’s the easiest way to multiply two fractions with models?

Start with unit fractions like \(\tfrac{1}{2}\times\tfrac{1}{3}\) — one row shaded, one column shaded, one overlap cell out of 6. Once that pattern is clear, move to non-unit fractions where you shade multiple rows and columns. The setup stays the same; just the counts change.

When do I use models to multiply fractions?

Use models when you’re first learning fraction multiplication, when a problem requires a visual explanation, or when you want to verify a numeric answer. After the multiplication rule feels automatic, models become a backup tool for proving the rule works.

Common mistakes when modeling fraction multiplication?

Drawing rows and columns both in the same direction (you need one horizontal, one vertical). Miscounting the overlap. Counting only the double-shaded part for the denominator (no — the denominator is ALL cells). Drawing uneven splits that make counting messy. Take a moment to draw straight, even lines.

How does the model compare to the multiplication rule?

The rule is faster: multiply numerators, multiply denominators. The model is slower but shows WHY the rule works — the overlapping region is literally one fraction OF the other. \(\tfrac{1}{2}\) of \(\tfrac{1}{3}\) means “half of one-third,” which the model shows as half of a third-strip. Same answer, two ways of seeing it.

Can I multiply two fractions with models without a calculator?

Yes. The whole process is paper-and-pencil drawing and counting. The numbers stay small. A ruler keeps the rectangle even, but it’s not required. No calculator at any step. Even the simplification at the end uses basic GCF skills.

Real-world examples of multiplying two fractions?

If \(\tfrac{2}{3}\) of the students in a class are girls and \(\tfrac{1}{2}\) of those girls play soccer, then \(\tfrac{1}{2}\times\tfrac{2}{3}=\tfrac{2}{6}=\tfrac{1}{3}\) of the class is a girl who plays soccer. If a road covers \(\tfrac{3}{4}\) mile and you’ve walked \(\tfrac{2}{3}\) of it, you’ve walked \(\tfrac{2}{3}\times\tfrac{3}{4}=\tfrac{1}{2}\) mile.

Worksheet for modeling fraction multiplication?

EffortlessMath has printable worksheets with pre-drawn grids for fraction multiplication models, plus blank graph-paper templates for self-drawing. The Grade 5 Math for Beginners workbook includes a full chapter on area-model fraction multiplication.

How to teach kids to multiply two fractions with models?

Start by folding a paper square in half horizontally — that’s the first fraction. Then fold in thirds vertically — that’s the second. Unfold and count the small rectangles created by the folds. Shade the overlap. The folding makes the abstract idea physical. Once they can model it, introduce the multiply-across rule and show it agrees.

Related EffortlessMath Lessons

If a topic on this page feels rusty, these short lessons go deeper:

• How to multiply fractions using models
• How to multiply fractions and whole numbers
• How to use arrays to multiply fractions by whole numbers
• How to divide unit fractions by whole numbers
• How to divide whole numbers by unit fractions