How to Solve the Complex Plane?
[include_netrun_products_block from-products="product/6-south-carolina-sc-ready-grade-3-math-practice-tests/" product-list-class="bundle-products float-left" product-item-class="float-left" product-item-image-container-class="p-0 float-left" product-item-image-container-size="col-2" product-item-image-container-custom-style="" product-item-container-size="" product-item-add-to-cart-class="btn-accent btn-purchase-ajax" product-item-button-custom-url="{{url}}/?ajax-add-to-cart={{id}}" product-item-button-custom-url-if-not-salable="{{productUrl}} product-item-container-class="" product-item-element-order="image,title,purchase,price" product-item-title-size="" product-item-title-wrapper-size="col-10" product-item-title-tag="h3" product-item-title-class="mt-0" product-item-title-wrapper-class="float-left pr-0" product-item-price-size="" product-item-purchase-size="" product-item-purchase-wrapper-size="" product-item-price-wrapper-class="pr-0 float-left" product-item-price-wrapper-size="col-10" product-item-read-more-text="" product-item-add-to-cart-text="" product-item-add-to-cart-custom-attribute="title='Purchase this book with single click'" product-item-thumbnail-size="290-380" show-details="false" show-excerpt="false" paginate="false" lazy-load="true"]
A complex number has both a real and an imaginary component. It’s the real part that has the number itself. The imaginary part, on the other hand, has the letter \(i\) attached to it. This imaginary \(i\) also has a mathematical definition. Imaginary number rule: \(?^2=−1\)
Related Topics
A step-by-step guide to graph the complex plane
Complex numbers cannot be plotted on a number line in the same way that real numbers can. We can still depict them graphically, though. To represent a complex number, we must consider both of its components.
As a way to represent the real and imaginary components of an object, we utilize a coordinate system known as a “complex plane”.
The complex numbers are positions on the plane represented as ordered pairs \((a, b)\), where \(a\) represents the horizontal axis coordinate and \(b\) represents the vertical axis coordinate.
- How to represent the components of a complex number on the complex plane?
- Calculate the real and imaginary parts of the complex number.
- Show the real component of the number by moving down the horizontal axis.
- To reveal the imaginary component of the number, move parallel to the vertical axis.
- Make a diagram of the spot.
The Complex Plane – Example 1:
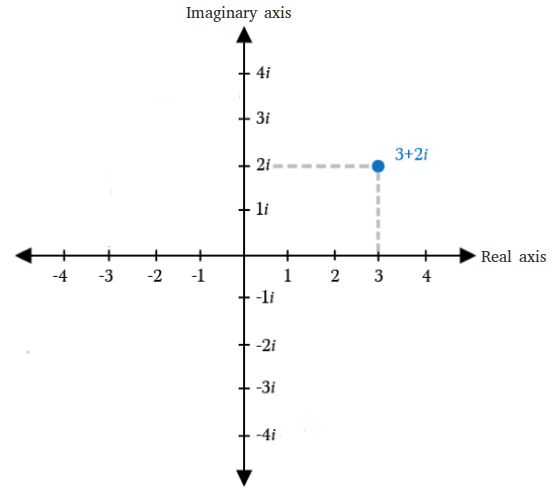
Plot the complex number \(3+2i\).
This number has a real part of \(3\) and an imaginary part of \(2\).
The Complex Plane – Example 2:
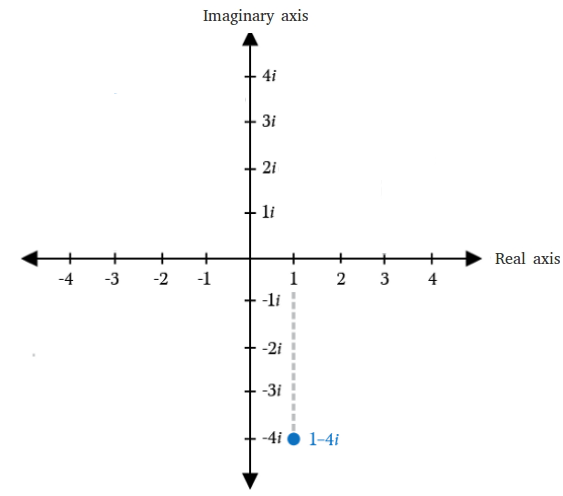
Plot the complex number \(1-4i\).
This number has a real part of \(1\) and an imaginary part of \(-4\).
Exercises for the Complex Plane
Graph these complex numbers.
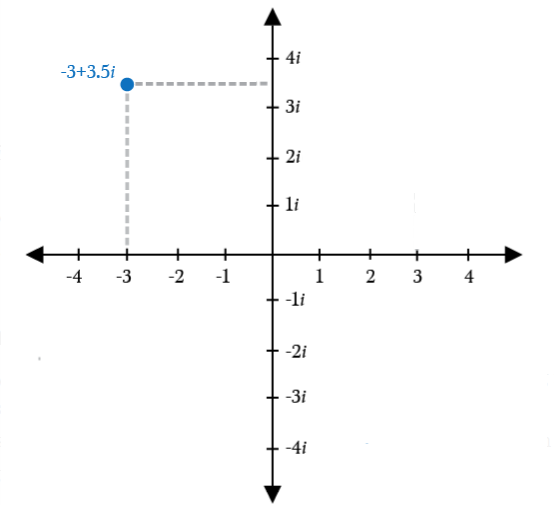
- \(\color{blue}{-3+3.5i}\)
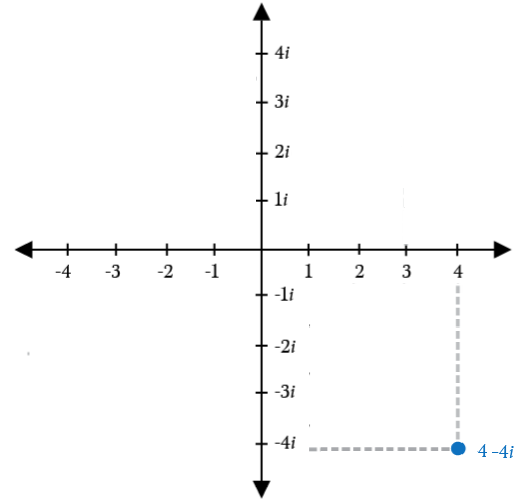
- \(\color{blue}{4-4i}\)
- \(\color{blue}{4-4i}\)
Related to This Article
More math articles
- The Ultimate 7th Grade NHSAS Math Course (+FREE Worksheets)
- 4th Grade WVGSA Math Worksheets: FREE & Printable
- How Is the ACCUPLACER Test Scored?
- GED Math Test-Taking Strategies
- How Does the Process of Renting Books from Online Bookstores Work? A Guide for Students
- 5th Grade Georgia Milestones Assessment System Math Practice Test Questions
- 4th Grade M-STEP Math Worksheets: FREE & Printable
- Battle of the Decimals: Using Grids for Easy Comparisons
- 7th Grade SBAC Math Worksheets: FREE & Printable
- Full-Length 8th Grade FSA Math Practice Test-Answers and Explanations
What people say about "How to Solve the Complex Plane? - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.