How to Master the Intricacies of the Coordinate Plane

Step-by-step Guide to Understand the Coordinate Plane

Here is a step-by-step guide to understand the Coordinate Plane:

Step 1: Diving into Origins – The Cartesian Theatre

Before delving into the crux of the matter, it’s enriching to appreciate the genesis of the coordinate plane. Descartes introduced this plane, painting a canvas where mathematics and geometry waltz harmoniously. It’s not just a grid; it’s the Cartesian plane, named after René Descartes.

Step 2: Grasping Basic Constructs – Axes and Quadrants

• Axes: Picture a cross in your mind. This is formed by two lines: the horizontal one, christened the \(x\)-axis, and the vertical counterpart, the \(y\)-axis.
• Quadrants: These axes, in their infinite wisdom, dissect the plane into four sections. Each of these is a quadrant. Starting from the top right and moving counterclockwise, we have the \(I, II, III\), and \(IV \)quadrants.

Step 3: Deciphering Coordinates – The Language of Points

A point on this plane is articulated as \((x, y)\). Here:

• \(x\): It whispers where the point lies along the horizontal axis.
• \(y\): It sings of the point’s position on the vertical axis.

Step 4: Plotting – An Art and Science

To craft a point on this canvas:

1. Start at the origin.
2. Go horizontally to the \(x\)-value.
3. Go vertically to meet the \(y\)-value.

Step 5: The Dance of Symmetry – Reflections and Rotations

The plane is a mirror. A point’s reflection across the \(x\)-axis or \(y\)-axis transmutes its coordinates. For instance, if you reflect a point across the \(x\)-axis, its \(y\)-coordinate pirouettes to its negative form.

Step 6: Beyond the 2D – Welcome to 3D and the z-axis

Imagine extruding our plane like a pop-up book. This introduces a third dimension, represented by the \(z\)-axis. Now, points dance in \(3D\) space with coordinates \((x, y, z)\).

Step 7: The Curve’s Whisper – Graphing Functions

Our plane isn’t just for points; it’s for stories. Functions weave these tales. For example, \(y = x²\) depicts a parabolic ballet, with every \(x\) pirouetting to its squared value in \(y\).

Step 8: Distances and Midpoints – The Hidden Relationships

Delve into the Pythagorean theorem and you can unveil the distance between two star-crossed points. Similarly, with a sprinkle of arithmetic, the midpoint formula unveils their secret meeting place.

Step 9: Slants and Slopes – Rise Over Run

Slope, a measure of steepness, is calculated as the rise (change in \(y\)) divided by the run (change in \(x\)). It’s the rhythm of linear functions, narrating how one variable ascends or descends relative to the other.

Step 10: Wrapping it Up – The Infinite Stories

As you stand on the precipice of this vast plane, know that it holds infinite tales. From linear tales to sinusoidal epics, the coordinate plane is more than a grid—it’s the universe of mathematical narratives. Dive deep, explore, and add your own story to this ever-expanding tapestry.

Example:

Find the coordinates of point \(A\).

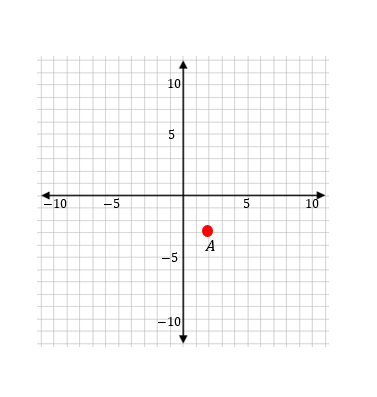
Solution:

Given a point on the coordinate plane, find its coordinates by determining its horizontal and vertical positions relative to the origin. The point \(A\) is located two units to the right and three units down from the origin have the coordinates \((2,-3)\).