How to Graphs of Rational Functions?

The rational function is defined as a polynomial coefficient whose denominator has a degree of at least (1). In other words, there must be a variable in the denominator.

The general form of a rational function is (frac{p(x)}{q(x)}). Where (p(x)) and (q(x)) are polynomials and (q(x)≠0).

Related Topics

• How to Add and Subtract Rational Expressions
• How to Multiply Rational Expressions
• How to Solve Rational Equations

A step-by-step guide to graphs of rational functions

It can be challenging to draw graphs of rational functions. Finding the asymptotes and intercepts is a fine place to begin when attempting to graph a rational function.

Graphing rational functions involves a series of steps:

• Determine if the rational function has any asymptotes
• Asymptotes should be drawn as dotted lines.
• Find the rational function’s (x) and (y)-intercepts, if any.
• Determine the (y) values for a variety of (x) values.
• Draw a smooth curve connecting the points by plotting them on a graph. Don’t cross-vertical asymptotes.

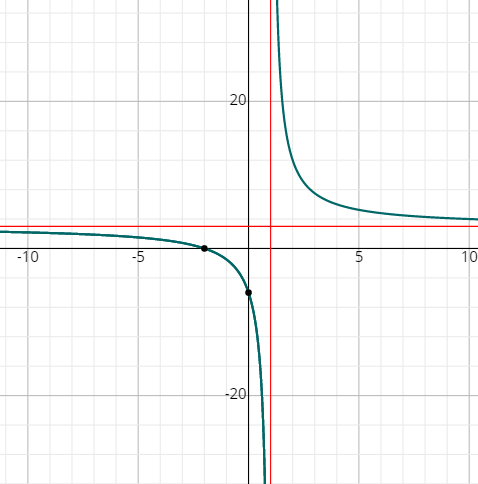
Graphs of Rational Functions, Example 1:

Draw a graph of the following function. (f(x)=frac{3x+6}{x-1})

First, we need to determine the vertical asymptotes:

(x-1=0 → x=1 )

And the horizontal asymptote is:

(y=frac{3}{1}=3)

Then, the (x)-intercepts will be,

(3x+6=0 → 3x=-6 → x=-frac{6}{3}=-2), the (x)-intercepts is ((-2,0))

The (y)-intercept is:

(f(0)=frac{3(0)+6}{0-1}) → (f(0)=frac{6}{-1}=-6), the (y)-intercepts is ((0,-6))

We need to find more points on the function and graph the function.

Now, putting all this together gives the following graph.

Exercises for Graphs of Rational Functions

Draw a graph of the following function.

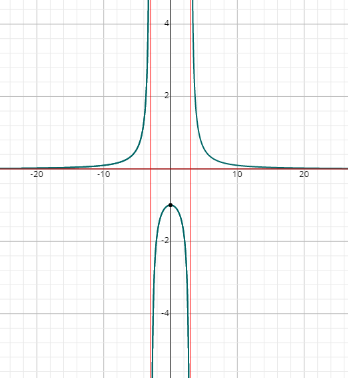
• (color{blue}{f(x)=frac{9}{x^2-9}})

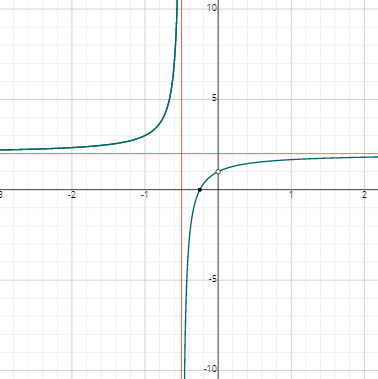
• (color{blue}{f(x)=frac{4x^2+x}{2x^2+x}})

• (color{blue}{f(x)=frac{x-2}{x^2-3x-4}})

(color{blue}{f(x)=frac{9}{x^2-9}})

(color{blue}{f(x)=frac{4x^2+x}{2x^2+x}})

(color{blue}{f(x)=frac{x-2}{x^2-3x-4}})