How to Find the Expected Value of a Random Variable?

The idea of expected value is a popular one in the world of finance. It’s a term used in the financial world to describe the potential future worth of an investment.

The \(EV\) of various scenarios may be calculated by calculating the odds of various probable outcomes.

Multivariate models and scenario analysis typically employ the notion. The expected return is directly related to this idea.

A step-by-step guide to the expected value of a random variable

The expected value formula:

The \(EV\) of a single event repeated several times are the first version of the expected value formula (think about tossing a coin). The formula for determining the \(EV\) in this situation is as follows:

\(\color{blue}{EV=P(X)\times n}\)

Where:

• \(EV\): the expected value
• \(P(X)\): the probability of the occurrence of the event
• \(n\):  the number of repetitions of the event

In finance, many problems related to the expected value involve several events. In such a scenario, the \(EV\) is the probability-weighted average of all possible events. Therefore, the general formula for finding \(EV\) for several events is as follows:

\(\color{blue}{EV=\sum P(X_i) \times X_i}\)

Where:

• \(EV\): the expected value
• \(P(X_i)\): the probability of the event
• \(X_i\):  the event

The Expected Value of a Random Variable – Example 1:

We were given the following discrete probability distribution:

What is the expected value?

To find the expected value, use this formula: \(\color{blue}{EV=\sum P(X_i) \times X_i}\)

\(EV=0 (\frac{1}{6})+ 1 (\frac{1}{6}) + 2 (\frac{1}{6})+ 3 (\frac{1}{6})\)

\(=0+\frac{1}{6}+\frac{2}{6}+\frac{3}{6}\) \(=\frac{0+1+2+3}{6}=\frac{6}{6}=1\)

\(EV=1\)

The Expected Value of a Random Variable – Example 2:

When you roll a die, you get paid \($2\) for an odd number and \($ 1\) for an even number. Find the expected value of money you get for one roll of the die.

The sample space of the experiment is:\((1,2,3,4,5,6)\)

First, we draw the table of the probability distribution for a single roll of a die and the amount paid for each result.

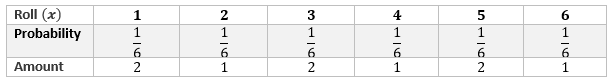
Then, to find the expected value, use this formula: \(\color{blue}{EV=\sum P(X_i) \times X_i}\)

\(EV= 2 (\frac{1}{6})+ 1 (\frac{1}{6})+ 2 (\frac{1}{6}) + 1 (\frac{1}{6})+ 2 (\frac{1}{6})+ 1 (\frac{1}{6})\)

\(=\frac{2}{6}+\frac{1}{6}+\frac{2}{6}+\frac{1}{6}+\frac{2}{6}+\frac{1}{6}\) \(=\frac{2+1+2+1+2+1}{6}\)=\(\frac{9}{6}\)

\(EV=\frac{9}{6}=1.5\)

Exercises for the Expected Value of a Random Variable

• A men’s soccer team plays zero football, one or two days a week. The probability that they will play on day zero is \(0.2\), the probability that they will play one day is \(0.5\) and the probability that they will play two days is \(0.4\). Find the expected value of the number of days per week the men’s soccer team plays football.

• A hospital researcher is interested in the number of times a typical patient calls the nurse after surgery during a \(12\)-hour shift. The following information was obtained from a random sample of \(40\) people. What is the expected value?

• In a city, \(12\%\) of families have three children, \(50\%\) of families have two children, \(22\%\) of families have one child, and \(11\%\) of families have no children. What is the expected value of children in a family?

• \(\color{blue}{1.3}\)
• \(\color{blue}{\frac{29}{10}}\)
• \(\color{blue}{1.58}\)