How to Find Transformation: Rotations, Reflections, and Translations?

Step by step guide to Find Transformation: Rotations, Reflections, and Translations

Transformation refers to the movement of objects in the coordinate plane. The transformation is an operation that maps the pre-image points to the image points without changing their size or shape.

Three types of transformation in the coordinate plane:

Reflection, Rotation, and Translation.

Reflection is flipping an object across a line without changing its size or shape. So, a reflection is a mirror image of the shape. In this case, the image is a reflection of the pre-image, and each point of the image is equidistant from each corresponding point in the pre-image.

Rotation is rotating an object about a fixed point without changing its size or shape. In this case, an image and its pre-image have the same shape and size, but the pre-image may be turned in different directions (for example, clockwise or counterclockwise).

The translation is sliding a figure in any direction without changing its size, shape, or orientation. In this case, a figure can be moved from one location in a coordinate plane to another location without changing its size, shape, or orientation.

Transformation: Rotations, Reflections, and Translations – Example 1:

Determine whether the given picture represents a reflection, rotation, or translation.

Solution:

The figure has been moved. So, the given movement represents a translation.

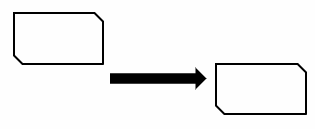
Transformation: Rotations, Reflections, and Translations – Example 2:

Determine whether the given picture represents a reflection, rotation, or translation.

Solution:

The figure has been flipped over the line. So, the given picture represents a reflection.

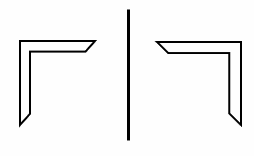
Transformation: Rotations, Reflections, and Translations – Example 3:

Determine whether the given picture represents a reflection, rotation, or translation.

Solution:

The figure has been rotated. So, the given picture represents a rotation.

Transformation: Rotations, Reflections, and Translations – Example 4:

Determine whether the given picture represents a reflection, rotation, or translation.

Solution:

The figure has been moved. So, the given movement represents a translation.

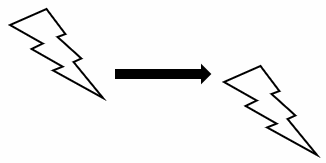
Exercises for Transformation: Rotations, Reflections, and Translations

Determine whether the given picture represents a reflection, rotation, or translation.

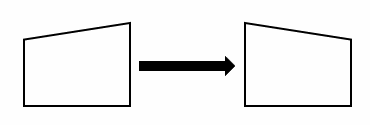
1.

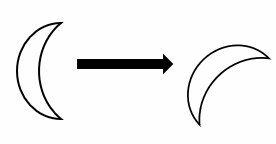
3.

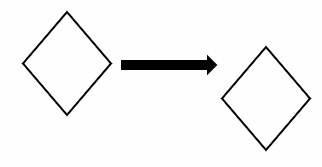
2.

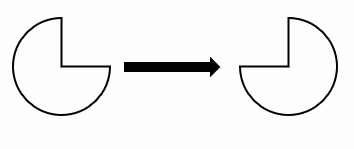
4.

1. rotation
2. reflection
3. translation
4. reflection