FREE SAT Math Practice Test

The Absolute Best Book to Ace the SAT Math Test

10 Sample SAT Math Practice Questions

1- A rectangle was altered by increasing its length by 20 percent and decreasing its width by s percent. If these alterations decreased the area of the rectangle by 4 percent, what is the value of s.

A. \(40\)

B. \(31\)

C. \(20\)

D. \(10\)

2- Tickets for a talent show cost $3 for children and $4 for adults. If John spends at least $10 but no more than $15 on \(x\) children tickets and 2 adult ticket, what are two possible values of \(x\)?

A. \(0, 1\)

B. \(1, 2\)

C. \(1, 4\)

D. \(1, 5\)

3- A function \(g(x)\) satisfies \(g(4)=5\) and \(g(5)=8\). A function \(f(x)\) satisfies f\((5)=18\) and
\(f(8)=32\). What is the value of \(f(g(5)\)?

A. \(12\)

B. \(22 \)

C. \(32\)

D. \(42\)

4- \(x^2+y^2+8x-2y=1\)
The equation of a circle in the \(xy\)-plane is shown above. What is the radius of the circle?

A. \(24\)

B. \(18\)

C. \(\sqrt{18}\)

D. \(\sqrt{10}\)

5- Right triangle ABC is shown below. Which of the following is true for all possible values of angle A and B?
\(\img{https://appmanager.effortlessmath.com/public/images/questions/satest.test1q45.jpg
}\)

A. \(tan A = tan B\)

B. \(sin A = cos B\)

C. \( tan^2 A=tan^2 B\)

D. \( tan A=1\)

6- \(y=cx^2+d , y=5\)
In the system of equations above, c and d are constants. For which of the following values of c and d does the system of equations have exactly two real solutions?

A. \( c = -2, d = 6\)

B.\( c =1, d = 7\)

C. \(c = – 3, d = 4\)

D. \(c = 5, d = 5\)

7- In five successive hours, a car travels 40 km, 45 km, 50 km, 35 km and 55 km. In the next five hours, it travels with an average speed of 50 km per hour. Find the total distance the car traveled in 10 hours.

A. \(425\) km

B. \(450\) km

C. \(475\) km

D. \(500\) km

8- In the \(xy\)-plane, the point \(4, 4\) lies on the graph of the function \(g(x)=4x^2+cx-10\). What is the value of c?

A. \(50\)

B. \(12.25\)

C. \(-2.5\)

D.\(-12.25\)

9- For what value of \(x\) is \(|x – 3| + 3\) equal to \(0\)?

A. \(1\)

B. \(2\)

C. no value of \(x\)

D. \(-3\)

10- \(\frac{c – d}{c}=a\)
In the equation above, if c is negative and d is positive, which of the following must be true?

A. \(a < 1\)

B.  \(a = 0\)

C. \(a > 1\)

D. \(a < -1\)

11- A restaurant sells salads for $5 each and drinks for $2 each. The restaurant’s revenue from selling a total of 300 salads and drinks in two days was $960. How many drinks were sold in total?__________

12- In the standard \((x,y)\) coordinate system plane, what is the area of the circle with the following equation? \(π=3.14\) (Round the answer to the whole number)__________
\(x^2+y^2+4x-8y+4=0\)

13- One of the zeros of the function \(f(x)=x^3-5x^2+6x\) is zero. If a and b are other zeros of this function and a is smaller than b, what is the value of a?__________

14- How many liters of a solution that is \(30\%\) salt must be added to 3 liters of a solution that is \(75\%\) salt to obtain a \(39\%\) salt solution?__________

15- Solve: \(f(x)=\frac{1}{(x-3)^2+4(x-3)+4}\)?__________

16- The sum of four numbers is \(600\). One of the numbers, \(x\) is \(50%\) more than the sum of the other three numbers. What is the value of \(x\)?__________

17- If \(f(x) = \frac{6x-6}{3}) and (f ^{–1}(x)\), is the inverse of \(f(x)\), what is the value of \(f ^{–1}(2)\)?__________

18- Two acute angles are shown above and \(cos⁡(a)=sin⁡(b)\). If \(a=3n+4\) and \(b=7n-10\), what is the value of n?__________
\(\img{https://appmanager.effortlessmath.com/public/images/questions/satest.test1q58.jpg
}\)

19- If \(8x-8=24\), what is the value of \(6x-4\)?

A. \(15\)

B. \(20\)

C. \(25\)

D. \(30\)

20- If \(f(x) = 3x + 4(x + 1) + 2\) then \(f(4x)=\)?

A. \(28x + 6\)

B. \(16x – 6\)

C. \(25x + 4\)

D. \(12x + 3\)

Best SAT Math Prep Resource for 2021

Answers:

2- B
Because each children ticket costs $3 and each adult ticket costs $4, the total amount, in dollars, that John spends on \(x\) student tickets and 2 adult ticket is \(3(x) + 4(2)\). Because John spends at least $10 but no more than $15 on the tickets, you can write the compound inequality \(3x + 8 ≥ 10\) and \(3x +8 ≤ 15.\)
Subtracting 8 from each side of both inequalities and then dividing each side of both inequalities by 3 gives \(x ≥ 0.66\) and \(x ≤ 2.3\).
Thus, the value of \(x\) must be an integer that is both greater than or equal to 0.66 and less than or equal to 2.3.
Therefore, \(x = 1\) or \(x = 2\). Either \(1\) or \(2\) may be gridded as the correct answer.

3- C
It is given that \(g(5)=8\). Therefore, to find the value of f\(g(5\)), substitute 8 for \(g(5)\).
\(f(g(5))=f(8)=32\)

4- C
The equation of a circle with center \((h, k)\) and radius r is \((x-h)^2+(y-k)^2=r^2\). To put the equation \( x^2+y^2+8x-2y=1\) in this form, complete the square as follows:
\(x^2+y^2+8x-2y=1\)
\((x^2+8x)+(y^2-2y)=1\)
\((x^2+8x+16)-16+(y^2-2y+1)-1=1\)
\((x+4)^2+(y-1)^2=18\)
\((x+4)^2+(y-1)^2=(\sqrt {18})^ 2\)
Therefore, the radius of the circle is \(\sqrt {18}\)

5- B
By definition, the sine of any acute angle is equal to the cosine of its complement.
Since, angle A and B are complementary angles, therefore:
\(sin A = cos B\)

6- A
Substituting 5 for y in \(y=cx^2+d\) gives \(5=cx^2+d\) which can be rewritten as \(5-d=cx^2\) .
Since y = 5 is one of the equations in the given system, any solution x of \(5-d=cx^2\) corresponds to the solution \((x,5)\) of the given system.
Since the square of a real number is always nonnegative, and a positive number has two square roots, the equation \(5-d=cx^2\) will have two solutions for \(x\) if and only if (1) c > 0 and d < 5 or (2) c < 0 and d >5.
Of the values for c and d given in the choices, only c\(=-2\), d=6 satisfy one of these pairs of conditions.
Alternatively, if c\(=-2\) and d=6, then the second equation would be
\(y=-2x^2+6 \)
The equation above has two real answer.

7- C
Add the first 5 numbers. \(40 + 45 + 50 + 35 + 55 = 225\)
To find the distance traveled in the next 5 hours, multiply the average by number of hours.
Distance = Average \(×\) Rate \(= 50 × 5 = 250\)
Add both numbers. 250 + 225 = 475

8- D
It is given that the function \(g(x)\) passes through the point (4, 4). Thus, if \(x=4\), the value of \(g(x)\) is 4 (since the graph of g in the \(xy\)-plane is the set of all points \(x,g(x)\).
Substituting 4 for \(x\) and 4 for g(x) in \(g(x)=4x^2+cx-10\) gives \(4 = 4(4)^2+c(4)-10\ )
Solve this equation for c. Then:
\( 4=64+4c-10\)
\(-50=4c→ c= -12.5\)

9- C
If the value of \(|x-3|+3\) is equal to 0, then \(|x-3|+3=0\).
Subtracting 3 from both sides of this equation gives \(|x-3|=-3\).
The expression \(|x – 3|\) on the left side of the equation is the absolute value of \(x – 3\), and the absolute value can never be a negative number.
Thus \(|x-3|=-3\) has no solution.
Therefore, there are no values for \(x\) for which the value of \(|x-3|+3\) is equal to\(0\).

10- C
The equation can be rewritten as
c – d = ac→(divide both sides by c) \(1-(\frac{d}{c})\) = a, since c < 0 and d > 0, the value of \(- (\frac{d}{c})\) is positive.
Therefore, 1 plus a positive number is positive. a must be greater than 1.
\(a > 1\)

11- 180
To determine the number of drinks sold, write and solve a system of two equations.
Let \(x\) be the number of salads sold and let \(y\) be the number of drinks sold. Then:
\(x+y=300\)
Since each salad cost \($5\), each drink cost \($2\), and the total revenue was \($960\), the equation
\(5x+2y=960\) is true.
The equation \(x+y=150\) is equivalent to \(2x+2y=600\), and subtracting each side of
\(2x+2y=600\) from the respective side of \(5x+2y=960\) gives \(3x=360\).
Therefore, the number of salads sold, x was x=120 and the number of drinks sold was:
\(y=300-120=180\)

12- 50
The equation of a circle with center (h, k) and radius r is:
\((x-h)^2+(y-k)^2=r^2\)
To put the equation \((x^2+y^2+4x-8y+4=0)\) in this form, complete the square as follows:
\(x^2+y^2+8x-8y+4=0\)
\(x^2+8x)+(y^2-8y)+4=0\)
\((x^2+4x+4)-4+(y^2-8y+16)-16+4=0\)
\((x+2)^2+(y-4)^2-20+4=0\)
\((x+2)^2+(y-4)^2-16=0\)
\((x+2)^2+(y-4)^2=16\)
\(r^2\) equals 16. Then, \(r=\sqrt {16}=4\)
The radius of the circle is 4. Thus, the area of the circle is:
\(A=πr^2=3.14(4)^2=3.14×16=50.24\)
Round the answer to whole number to get \(50\).

13- 2
First factor the function:
\( f(x)=x^3-5x^2+6x=x (x-2)(x-3)\)
To find the zeros, \(f(x)\) should be zero.
\(f(x)=x (x-2)(x-3)=0\)
Therefore, the zeros are:
\( x=0\)
\((x-2)=0 ⇒ x=2\)
\((x-3)=0 ⇒ x=3\)

14- 12
Let \(x\) represent the number of liters of the \(30\%\) solution.
The amount of salt in the \(30\%\) solution \(0.30x\) plus the amount of salt in the \(75\%\) solution \((0.75) × (5)\) must be equal to the amount of salt in the \(39\%\) mixture \(0.39 × (x + 5)\). Write the equation and solve for \(x\).
\( 0.30x+0.75(3)=0.39(x+3)→0.30x+2.25=0.39x+1.17→\)
\( 0.39x-0.30x=2.25-1.17→0.09x=1.08→x=\frac{1.08}{0.09} = 12\)

15- 1
The function \(f(x)\) is undefined when the denominator of
\(\frac{1}{(x-3)^2+4(x-3)+4}\)
is equal to zero. The expression \((x-3)^2+4(x-3)+4\) is a perfect square.
\((x-3)^2+4(x-3)+4=((x-3)+2)^2\) which can be rewritten as \((x-1)^2\). The expression \((x-1)^2\ ) is equal to zero if and only if \(x=1\). Therefore, the value of x for which \(f(x)\) is undefined is \(1\).

16- 360
One of the four numbers is \(x\); let the other three numbers be \(y, z\) and w. Since the sum of four numbers is 600, the equation \(x + y + z+w = 600\) is true. The statement that x is \(50%\) more than the sum of the other three numbers can be represented as:
\(x = 1.5(y + z+w) \space or \space \frac{x}{1.5}=y+z+w→\frac{2x}{3}=y+z+w\)
Substituting the value \(y+z+w\) in the equation \(x + y + z + w = 600\)
\(gives \space x+\frac{2x}{3}=600→\frac{5x}{3}=600→5x=1,800→x=\frac{1800}{5}=360\)

17- 2
To solve for the inverse function, first replace \(f(x)\) with y. Then, solve the equation for \(x\) and after that replace every \(x\) with a y and replace every y with an x. Finally, replace y with \(f^{-1 }(x)\).
\(f(x)=\frac{6x-6}{3}⇒ y=\frac{6x-6}{3}⇒ 3y = 6x –6 ⇒3y+6=6x⇒\frac{3y + 6}{6}=x\)
\(f ^ {-1}(x)=\frac{3x + 6}{6}⇒ f^{-1} (2)=\frac{3(2)+6}{6}=\frac{12}{6}=2\)

18- 9.6
Since the angles are acute and \( sin(a^\circ)=cos(b^\circ)\), the angles are complementary angles and \(a+b=90\).
Substituting \(3n+4\) for a and \(7n-10\) for b gives\(,(3n+4)+(7n-10) = 90\)
which simplifies to\( 10n – 6 = 90\). Therefore, \(10n = 96\), and \(n = 9.6\)

19- B
Add 8 both sides of the equation \(8x – 8 = 24\) gives \(8x = 24 + 8 = 32\).
Dividing each side of the equation \(8x = 32 \)by \(8\) gives \(x = 4\).
Substituting 4 for \(x\) in the expression \(6x – 4\) gives \(6(4) – 4 = 20\).

20- A
If \(f(x) = 3x + 4(x+1)+2\), then find \(f(4x)\) by substituting \(4x\) for every \(x\) in the function. This gives: \( f(4x) = 3 (4x) + 4(4x+1)+2\), It simplifies to: \(f(4x)= 3 (4x)+ 4(4x+1)+2=12x+16x+4+2=28x+6\)

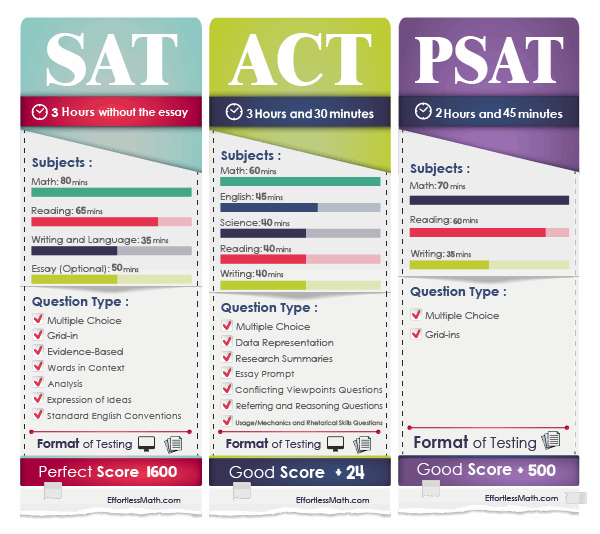
College Entrance Tests

Looking for the best resource to help you succeed on the SAT Math test?

The Best Books to Ace the SAT Math Test