Distinguishing Angles: Acute, Right, Obtuse, and Straight
In this article, we want to provide you with information about angles and their types.

Angles get created whenever \(2\) lines overlap at a point. Then, the measure of the ‘opening’ in-between those \(2\) rays is known as an ‘angle’. It’s shown via the symbol \(∠\).
Angles normally get measured via degrees and radians, and this is a measure of circularity or rotation.
We see angles every day as engineers, as well as architects, utilize angles to design buildings, roads, as well as sporting facilities.
There are \(2\) main components associated with angles – the arms and the vertex.
Related Topics
Angle Arms
The \(2\) rays that link up at a common point and create an angle are known as angle arms.
Vertex of an angle
Vertex is a commonplace endpoint shared by the \(2\) rays.
Angle measurement
Angles get measured in degrees. One complete rotation around a point creates a complete angle of \(360°\).
Kinds of Angles and Properties
Six kinds of angles exist. Each one comes with its specific identification of the source of angle measurement. Let’s find out about each one of these kinds of angles separately along with the properties they have.
Acute Angles
Acute angles are angles with a measurement bigger greater than \(0°\) and lower than \(90°\).
Right Angles
Whenever an angle measures \(90°\), it’s called a right angle. Right angles can be easily known since they form the shape of the letter \(L\).
Obtuse Angles
Whenever an angle measures lower than \(180°\) yet bigger than \(90°\) it’s called an obtuse angle.
Straight Angles
Angles created via straight lines are called straight angles. Straight angles are made with straight lines, and an angle created in-between \(2\) rays are equivalent to \(180°\). With straight angles, the \(2\) rays are opposite of one another. Two right angles create a straight angle. Because the measurement of a straight angle is \(180°\), it’s one-half of the complete turn of a circle.
Reflex Angles
Reflex angles are angles that measure more than \(180°\) yet lower than \(360°\).
Complete Angles
Whenever an angle’s measurement is equivalent to \(360°\) it’s a complete angle.
Missing Numerals – Example 1:
Write the name of the angle.
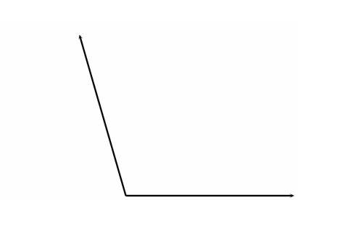
Solution:
This angle measures lower than \(180°\) yet bigger than \(90°\). So, it’s an obtuse angle.
Missing Numerals – Example 2:
Write the name of the angle.
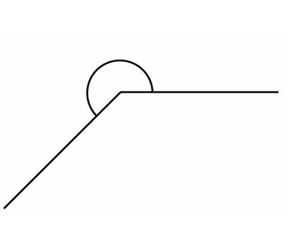
Solution:
This angle measure more than \(180°\) yetis lower than \(360°\). So, it’s a Reflex angles.
Exercises for Missing Numerals
Write the name of the angles.
1)
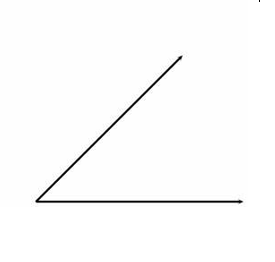
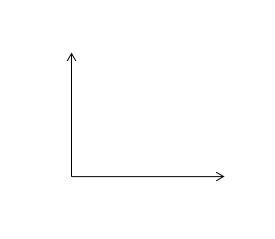
2)

- \(\color{blue}{Acute}\)
- \(\color{blue}{Right}\)
Related to This Article
More math articles
- 4th Grade Scantron Math Worksheets: FREE & Printable
- 6th Grade PARCC Math Worksheets: FREE & Printable
- FREE 7th Grade FSA Math Practice Test
- Ratio, Proportion and Percentages Puzzle -Critical Thinking 9
- How to Solve Rational Exponents and Radicals?
- How to Find Interval Notation
- How to Find Complementary and Supplementary Angles? (+FREE Worksheet!)
- The Ultimate PERT Math Course (+FREE Worksheets & Tests)
- How to Do Division Using Repeated Subtraction
- SAT Math Level 2 Calculator Tips to Boost Your Score


























What people say about "Distinguishing Angles: Acute, Right, Obtuse, and Straight - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.