Area of a Trapezoid
In this article, you will gain a comprehensive understanding of what a trapezoid is and how to calculate its area using the trapezoid area formula.
A step-by-step guide to finding the area of a trapezoid
To determine the area of a trapezoid, follow these simple steps:
Step 1: Multiply the sum of the bases (the parallel sides) by the height (the perpendicular distance between the bases)
Step 2: Divide the product by 2.
So, the area of a trapezoid\( =\frac{1}{2}×\)(Sum of parallel sides)\(×\)(perpendicular distance in between the parallel sides).
Definition of the Area of a Trapezoid – Example 1
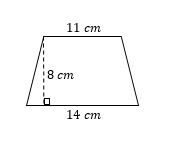
What is the area of this trapezoid?
Solution:
According to the area of a trapezoid:
Step 1: Add the parallel sides. \(14+11=25\)
Step 2: Multiply it by the perpendicular distance between the parallel sides. \(25×8=200\)
Step 3: Multiply the product by \(\frac{1}{2}\).
\(\frac{1}{2}×200=100\). So, the area of this trapezoid is \(100 cm^2\).
Definition of the Area of a Trapezoid – Example 2
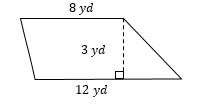
What is the area of this trapezoid?
Solution:
According to the area of a trapezoid:
Step 1: Add the parallel sides. \(12+8=20\)
Step 2: Multiply it by the perpendicular distance between the parallel sides. \(20×3=60\)
Step 3: Multiply the product by \(\frac{1}{2}\).
\(\frac{1}{2}×60=30\). So, the area of this trapezoid is \(60 yd^2\).
Related to This Article
More math articles
- Top 10 8th Grade PARCC Math Practice Questions
- How To Choose the Right DAT Course?
- How to Solve Word Problems Involving Comparing Percent and Fractions?
- 3rd Grade North Carolina End-of-Grade Math Worksheets: FREE & Printable
- 4th Grade PARCC Math Practice Test Questions
- How to Choose the Right Visual: Models for Multiplying Fractions by Whole Numbers
- 4th Grade M-STEP Math Worksheets: FREE & Printable
- How to Find the Reciprocal Trigonometric Functions?
- The Ultimate ACT Math Course (+FREE Worksheets & Tests)
- Top 10 Tips to Create an ISEE Math Study Plan

















What people say about "Area of a Trapezoid - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.