Area of a Parallelogram
The primary goal of this article is to provide a comprehensive guide that will enable you to understand and apply the definition of the area of a parallelogram.

A step-by-step guide to finding the area of a parallelogram
A parallelogram is a quadrilateral that has two pairs of parallel sides.
The formula for understanding the area of a parallelogram is base times height.
It means A\(=\)bh. This formula is identical to the formula for the area of a rectangle.
The opposite sides of the parallelogram are identical in length.
The opposite angles of the parallelogram are identical in measure.
The sum of all the interior angles in a parallelogram is equal to 360 degrees.
Definition of the Area of a Parallelogram – Example 1
What is the area of this parallelogram?
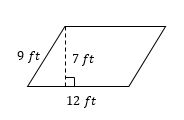
Solution:
To determine the area of a parallelogram:
Multiply the base by the height. \(12×7=84\)
So, the area of a parallelogram is \(84 ft^2\).
Area\(=\)base\(×\)height\(→A=b×h→A=12×7=84\)
Definition of the Area of a Parallelogram – Example 2
What is the area of this parallelogram?
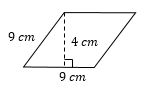
Solution:
To determine the area of a parallelogram:
Multiply the base by the height. \(9×4=36\)
So, the area of a parallelogram is \(36 cm^2\).
Area\(=\)base\(×\)height→\(A=b×h→A=9×4=36\)
Related to This Article
More math articles
- Top 10 HSPT Math Practice Questions
- Addition of 3-Digit Numbers
- Place Values Relationship
- How to Construct the Incircle of a Triangle
- Can the CBEST Test be Waived?
- The Ultimate ASTB Math Course (+FREE Worksheets & Tests)
- 4th Grade MCAP Math Worksheets: FREE & Printable
- How to Order Fractions: Step-by-Step Guide
- How is the TExES Core Subjects Scored?
- 7th Grade PSSA Math FREE Sample Practice Questions


































What people say about "Area of a Parallelogram - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.