10 Most Common Pre-Algebra Math Questions
Preparing for the Pre-Algebra Math test? Want a preview of the most common mathematics questions on the Pre-Algebra Math test? If so, then you are in the right place.
The mathematics section of Pre-Algebra can be a challenging area for many test-takers, but with enough patience, it can be easy and even enjoyable!
Preparing for the Pre-Algebra Math test can be a nerve-wracking experience. Learning more about what you’re going to see when you take the Pre-Algebra can help to reduce those pre-test jitters. Here’s your chance to review the 10 most common Pre-Algebra Math questions to help you know what to expect and what to practice most. Try these 10 most common Pre-Algebra Math questions to hone your mathematical skills and to see if your math skills are up to date on what’s being asked on the exam or if you still need more practice.
Make sure to follow some of the related links at the bottom of this post to get a better idea of what kind of mathematics questions you need to practice.
The Absolute Best Book to Ace the Pre-Algebra
10 Sample Pre-Algebra Math Practice Questions
1- Simplify \(6x^2 y^3 (2x^2 y)^3= \)
A. \(12x^4 y^6\)
B. \(12x^8 y^6\)
C. \(48x^4 y^6\)
D. \(48x^8 y^6\)
2- The diagonal of a rectangle is \(10\) inches long and the height of the rectangle is \(8\) inches. What is the perimeter of the rectangle in inches?______
3-What is the value of \(x\) in the following equation?
\(\frac{2}{3} x+\frac{1}{6}= \frac{1}{3} \)
A. 6
B. \(\frac{1}{2}\)
C. \(\frac{1}{3}\)
D. \(\frac{1}{4}\)
4- A card is drawn at random from a standard \(52\)–card deck, what is the probability that the card is of Hearts? (The deck includes \(13\) of each suit clubs, diamonds, hearts, and spades)
A. \(\frac{1}{3}\)
B. \(\frac{1}{6}\)
C. \(\frac{1}{52}\)
D. \(\frac{1}{4}\)
5- Which of the following shows the numbers in ascending order?
\(\frac{2}{3} , 0.68 , 67\% , \frac{4}{5}\)
A. \(67\%\), 0.68, \(\frac{2}{3}\),\(\frac{4}{5}\)
B. \(67\%\), 0.68, \(\frac{4}{5} \), \(\frac{2}{3}\)
C. 0.68, \(67\%\), \(\frac{2}{3} \), \(\frac{4}{5}\)
D. \(\frac{2}{3}\), \(67\%\), 0.68,\(\frac{4}{5}\)
6- The mean of \(50\) test scores was calculated as \(88\). But, it turned out that one of the scores was misread as \(94\) but it was \(69\). What is the mean?
A. \(85\)
B. \(87\)
C. \(87.5\)
D. \(88.5\\)
7- Two dice are thrown simultaneously, what is the probability of getting a sum of \(6\) or \(9\)?
A. \(\frac{1}{3}\)
B. \(\frac{1}{4}\)
C. \(\frac{1}{6}\)
D. \(\frac{1}{2}\)
8- Jason is \(9\) miles ahead of Joe running at \(5.5\) miles per hour and Joe is running at the speed of \(7\) miles per hour. How long does it take Joe to catch Jason?
A. \(3\) hours
B. \(4\) hours
C. \(6\) hours
D. \(8\) hours
9- \(55\) students took an exam and \(11\) of them failed. What percent of the students passed the exam?
A. \(40\%\)
B. \(60\%\)
C. \(80\%\)
D. \(20\%\)
10- What is the volume of a box with the following dimensions?
Height \(= 4 cm\), Width \(= 5 cm\), Length \(= 6 cm\)
A. \(15 cm^3\)
B. \(60 cm^3\)
C. \(90 cm^3\)
D. \(120 cm^3\)
Best Algebra Prep Resource
Answers:
1- D
Simplify.
\(6x^2 y^3 (2x^ 2 y)^ 3= 6x^ 2 y^ 3 (8x^ 6 y^ 3 ) = 48x^ 8 y^ 6\)
2- 28
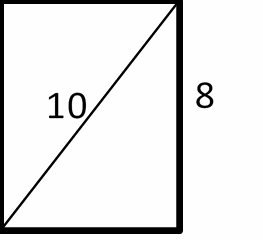
Let \(x\) be the width of the rectangle. Use Pythagorean Theorem:
\(a^ 2 + b^ 2 = c^ 2\)
\(x^ 2 + 8^ 2 = 10^ 2 {\Rightarrow} x^ 2 + 64 = 100 {\Rightarrow} x^ 2 = 100 – 64 = 36 ⇒ x = 6\)
Perimeter of the rectangle \(= 2 (length + width) = 2 (8 + 6) = 2 (14) = 28\)
3- D
Isolate and solve for \(x\).
\(\frac{2}{3} x+\frac{1}{6} = \frac{1}{3} {\Rightarrow} \frac{2}{3} x= \frac{1}{3} -\frac{1}{6} = \frac{1}{6} {\Rightarrow} \frac{2}{3} x= \frac{1}{6}\)
Multiply both sides by the reciprocal of the coefficient of \(x\).
\((\frac{3}{2}) \frac{2}{3} x= \frac{1}{6} (\frac{3}{2}) {\Rightarrow} x= \frac{3}{12}=\frac{1}{4}\)
4- D
The probability of choosing a Hearts is \(\frac{13}{52}=\frac{1}{4}\)
5- D
Change the numbers to decimal and then compare.
\(\frac{2}{3} = 0.666… \)
\(0.68 \)
\(67\% = 0.67\)
\(\frac{4}{5} = 0.80\)
Therefore
\(\frac{2}{3} < 67\% < 0.68 < \frac{4}{5}\)
6- C
average (mean) =\(\frac{(sum \space of \space terms)}{(number \space of \space terms)} {\Rightarrow} 88 = \frac{(sum \space of \space terms)}{50} {\Rightarrow} sum = 88 {\times} 50 = 4400\)
The difference of \(94\) and \(69\) is \(25\). Therefore, \(25\) should be subtracted from the sum.
\(4400 – 25 = 4375\)
mean \(=\) \(\frac{(sum of terms)}{(number of terms)}\) ⇒ mean \(=\) \(\frac{(4375)}{50}= 87.5\)
7- B
To get a sum of \(6\) for two dice, we can get \(5\) different options:
\((5, 1), (4, 2), (3, 3), (2, 4), (1, 5)\)
To get a sum of \(9\) for two dice, we can get \(4\) different options:
\((6, 3), (5, 4), (4, 5), (3, 6)\)
Therefore, there are \(9\) options to get the sum of \(6\) or \(9\).
Since we have \(6 × 6 = 36\) total options, the probability of getting a sum of \(6\) and \(9\) is \(9\) out of \(36\) or \(\frac{1}{4}\).
8- C
The distance between Jason and Joe is \(9\) miles. Jason running at \(5.5\) miles per hour and Joe is running at the speed of \(7\) miles per hour. Therefore, every hour the distance is \(1.5\) miles less. \(9 \div 1.5 = 6\)
9- C
The failing rate is \(11\) out of \(55\) = \(\frac{11}{55} \)
Change the fraction to percent:
\(\frac{11}{55} {\times} 100\%=20\% \)
20 percent of students failed. Therefore, \(80\) percent of students passed the exam.
10- D
Volume of a box = length \(\times \) width \(\times \) height \(= 4 \times 5 \times 6 = 120\)
The Best Books to Ace Algebra
Related to This Article
More math articles
- Best Calculator For 11th Grade Students
- 4th Grade STAAR Math FREE Sample Practice Questions
- TABE Math FREE Sample Practice Questions
- Calculating the Surface Area of Prisms and Cylinders
- 5th Grade LEAP Math Worksheets: FREE & Printable
- Word Problems: Fractions
- Travel-Friendly Teaching Supplies for A Portable Classroom
- 10 Most Common SIFT Math Questions
- AFOQT Math Formulas
- A Comprehensive Collection of Free PERT Math Practice Tests
What people say about "10 Most Common Pre-Algebra Math Questions - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.