Algebra Puzzle – Challenge 42
Enjoy solving mathematical and critical thinking challenges? This is a great math puzzle for those who love math challenges!
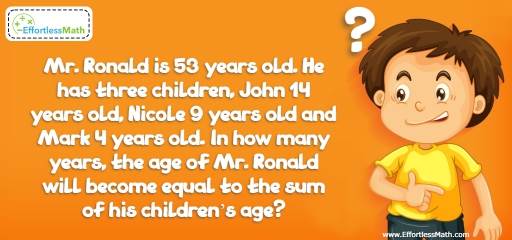
Challenge:
Mr. Ronald is 53 years old. He has three children, John 14 years old, Nicole 9 years old, and Mark 4 years old. In how many years, the age of Mr. Ronald will become equal to the sum of his children’s age?
The Absolute Best Book to Challenge Your Smart Student!
The correct answer is 13.
Mr. Ronald is 53 years old and the sum of his children is:
14 + 9 + 4 = 27
The difference of Mr. Ronald’s age and his children is:
53 – 27 = 26
Every year, one year adds to Mr. Ronald’s age and three years adds to the sum of his children’s age. The difference of this is 2 years. So, each year 2 years is subtracted from the difference of Mr. Ronal’s age and his children.
Therefore, in 13 years the difference of Mr. Ronald’s age and his children becomes zero.
The Absolute Best Books to Ace Algebra
Related to This Article
More math articles
- Why do I Struggle with Math so much?
- How to Prepare for the TSI Math Test?
- How to Break Fractions and Mixed Numbers Apart to Add or Subtract
- Top 10 7th Grade FSA Math Practice Questions
- Top 10 TSI Math Prep Books (Our 2023 Favorite Picks)
- 10 Most Common 5th Grade Georgia Milestones Assessment System Math Questions
- 3rd Grade OST Math Practice Test Questions
- The Ultimate ILTS Elementary Education Math (Grades 1–6) (305) Course
- Top 10 Free Websites for AFOQT Math Preparation
- How to Distinguishing Numbers: Prime vs. Composite

















What people say about "Algebra Puzzle – Challenge 42 - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.